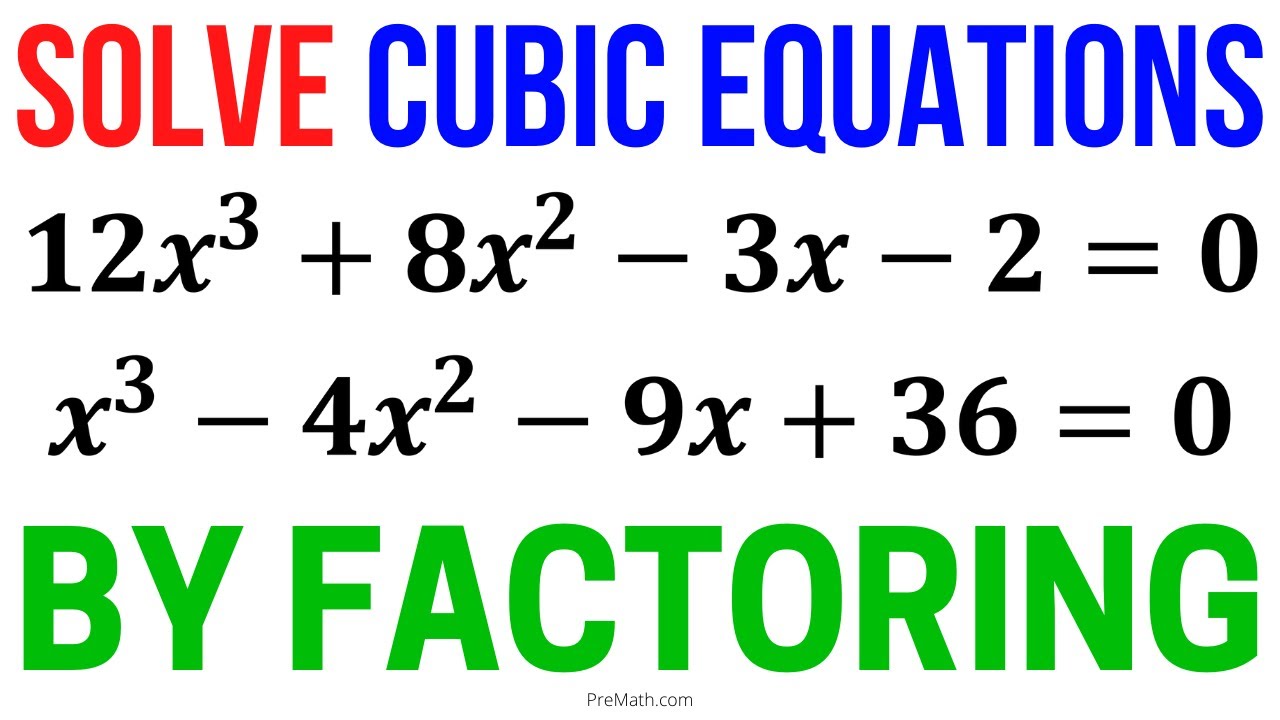1 Group the polynomial into two sections. Grouping the polynomial into two sections will let you attack each section individually. [1] Say we're working with the polynomial x 3 + 3x 2 - 6x - 18 = 0. Let's group it into (x 3 + 3x 2) and (- 6x - 18) 2 Find what's the common in each section. Looking at (x 3 + 3x 2 ), we can see that x 2 is common. What does it mean to factorize a cubic polynomial? In math, the factors of any polynomial represent components or "building blocks" of the polynomial. Whenever you factor a polynomial (cubic or otherwise), you are finding simpler polynomials whose product equals the original polynomial.

How to factor a cubic function YouTube
To factorise a cubic, you need the value of b (and sometimes a ). To find this factor, use the factor theorem. The factor theorem says that, for a function f ( x ): if is a factor, the ; if is a factor then . For example, the cubic below has a factor of : You can show this by calculating f (-2); if ( x + 2) really is a factor, f (-2) will equal 0: Factoring cubic polynomials is a process of expressing the cubic polynomials as a product of their factors. We can find the factors of a cubic polynomial using long division methods, algebraic identities, grouping, etc. A cubic polynomial has a standard form ax 3 + bx 2 + cx + d, where a, b, c, d are real numbers. A cubic polynomial is a polynomial of the form f (x)=ax^3+bx^2+cx+d, f (x) = ax3 +bx2 +cx+ d, where a\ne 0. a = 0. If the coefficients are real numbers, the polynomial must factor as the product of a linear polynomial and a quadratic polynomial. Contents Existence of a Linear Factor Factoring in Practice Factoring - General Case How to factor a cubic function Cowan Academy 82.6K subscribers Join Subscribe Subscribed 457K views 6 years ago Learn the steps on how to factor a cubic function using both rational roots.

How to Factor a Cubic Polynomial 12 Steps (with Pictures)
Factorization aims to represent a polynomial as a product of two (or more) lower degree polynomials. The factorization is complete when the resulting factors are irreducible. The Standard Form of Cubic Polynomial As we are focused on the cubic polynomial, we need its standard form for our discussion. Standard form of the Cubic Polynomial A cubic polynomial is of the form p(x) = a 3x3 + a 2x2 + a 1x+ a 0: The Fundamental Theorem of Algebra guarantees that if a 0;a 1;a 2;a 3 are all real numbers, then we can factor my polynomial into the form p(x) = a 3(x b 1)(x2 + b 2c+ b 3): In other words, I can always factor my cubic polynomial into the product of a rst degree polynomial A general cubic equation is of the form (1) (the coefficient of may be taken as 1 without loss of generality by dividing the entire equation through by ). The Wolfram Language can solve cubic equations exactly using the built-in command Solve [ a3 x^3 + a2 x^2 + a1 x + a0 == 0, x ]. This algebra 2 and precalculus video tutorial explains how to factor cubic polynomials by factoring by grouping method or by listing the possible rational zeros of the polynomial and then by.
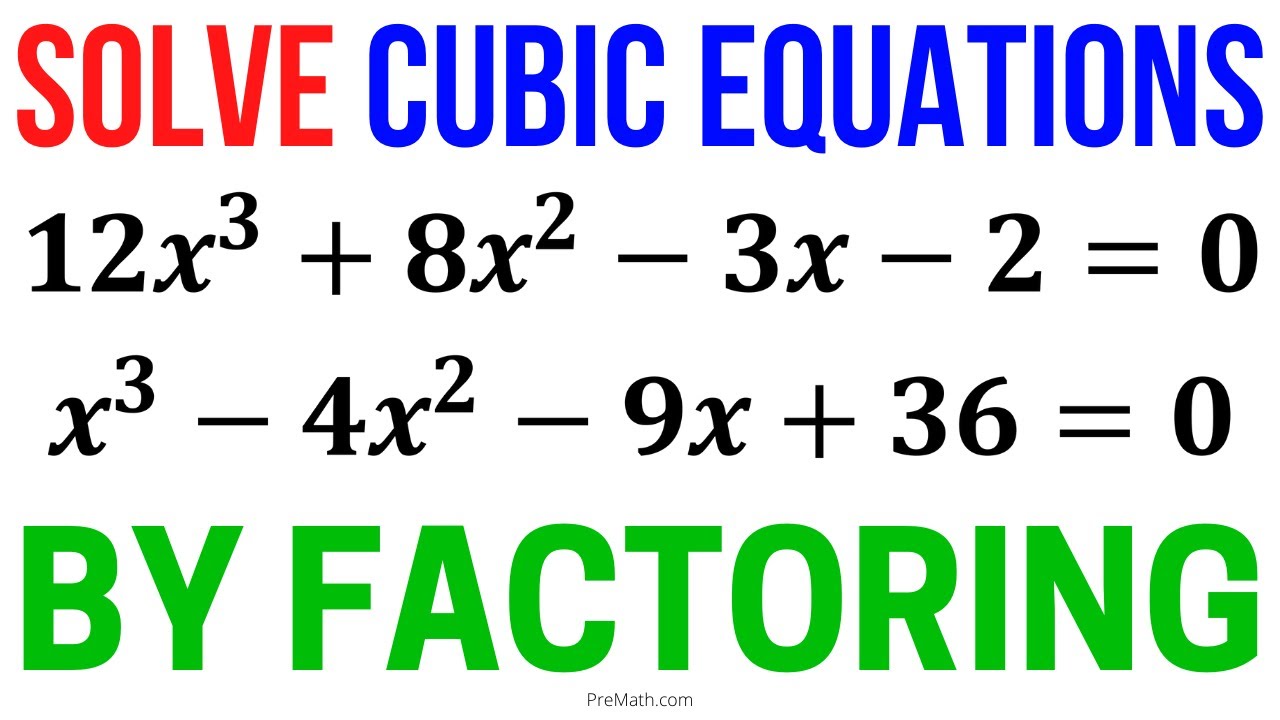
Learn How to Solve Cubic Equations FAST Easy Factoring Method StepbyStep Tutorial YouTube
About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How YouTube works Test new features NFL Sunday Ticket Press Copyright. Factorising Cubic Polynomial Last updated at May 29, 2023 by Teachoo To factorise cubic polynomial p (x), we Find x = a where p (a) = 0 Then (x - a) is the factor of p (x) Now divide p (x) by (x - a) i.e. (p (x))/ ( (x - a)) And then we factorise the quotient by splitting the middle term Tired of ads?
Full pad Examples Frequently Asked Questions (FAQ) What is the sum of cubes formula? The sum of cubes formula is a³ + b³ = (a+b) (a² - ab + b²) What is the difference of squares formula? The difference of squares formula is a² - b² = (a+b) (a-b) What is the difference of cubes formula? Zero is the easiest to check because that would mean the constant term is zero, thats not the case here. 1 doesn't work because that would be 1 − 6 + 4 = −1 ≠ 0. 2 happens to work since this would be 23 − 6 ⋅ 2 + 4 = 8 − 12 + 4 = 0. Since 2 works, we know that the equation can be factored as (x − 2)q(x) where q(x) = x3−6x+4 x−2.
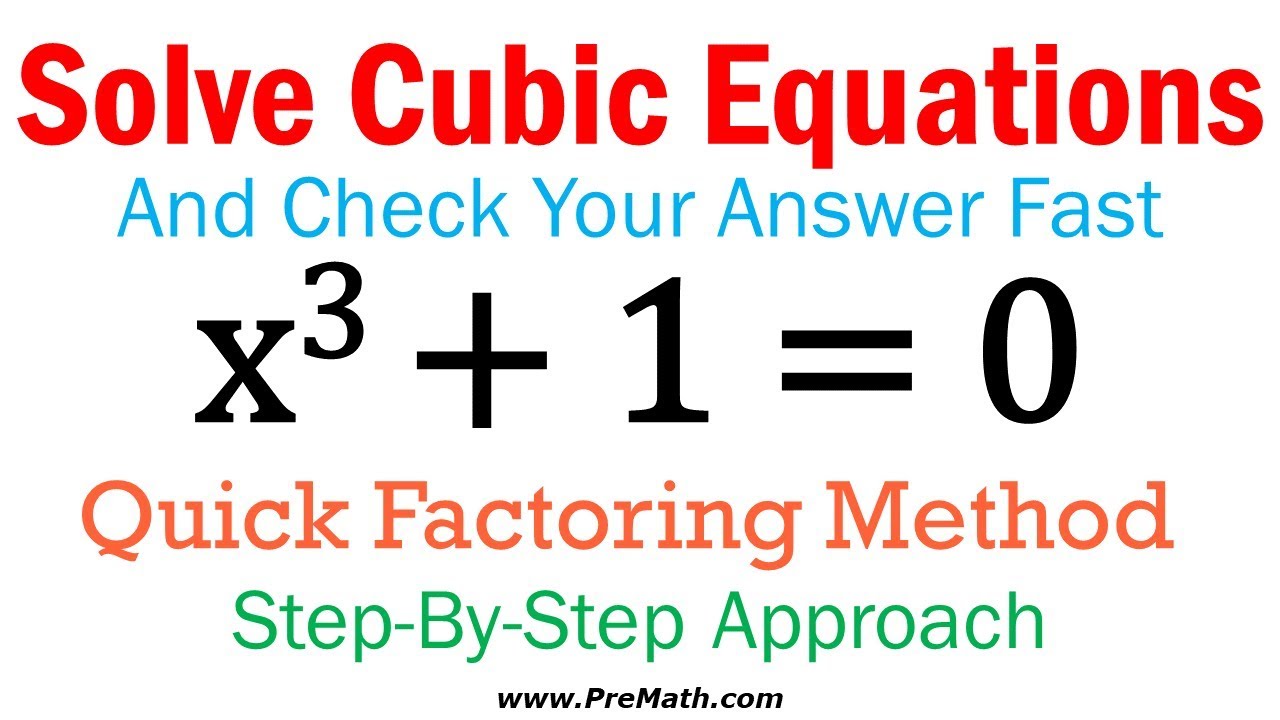
Solve Cubic Equations The Factoring Method YouTube
How to factorise a cubic polynomial (Version 1) : ExamSolutions This tutorial shows you how to factorise a given cubic polynomial by using the factor theorem and algebraic long division. Example: Factorise 2x 3 - 3x 2 - 11x + 6. Show Step-by-step Solutions Use this calculator to solve polynomial equations with an order of 3 such as ax3 + bx2 + cx + d = 0 for x including complex solutions. Enter positive or negative values for a, b, c and d and the calculator will find all solutions for x. Enter 0 if that term is not present in your cubic equation.