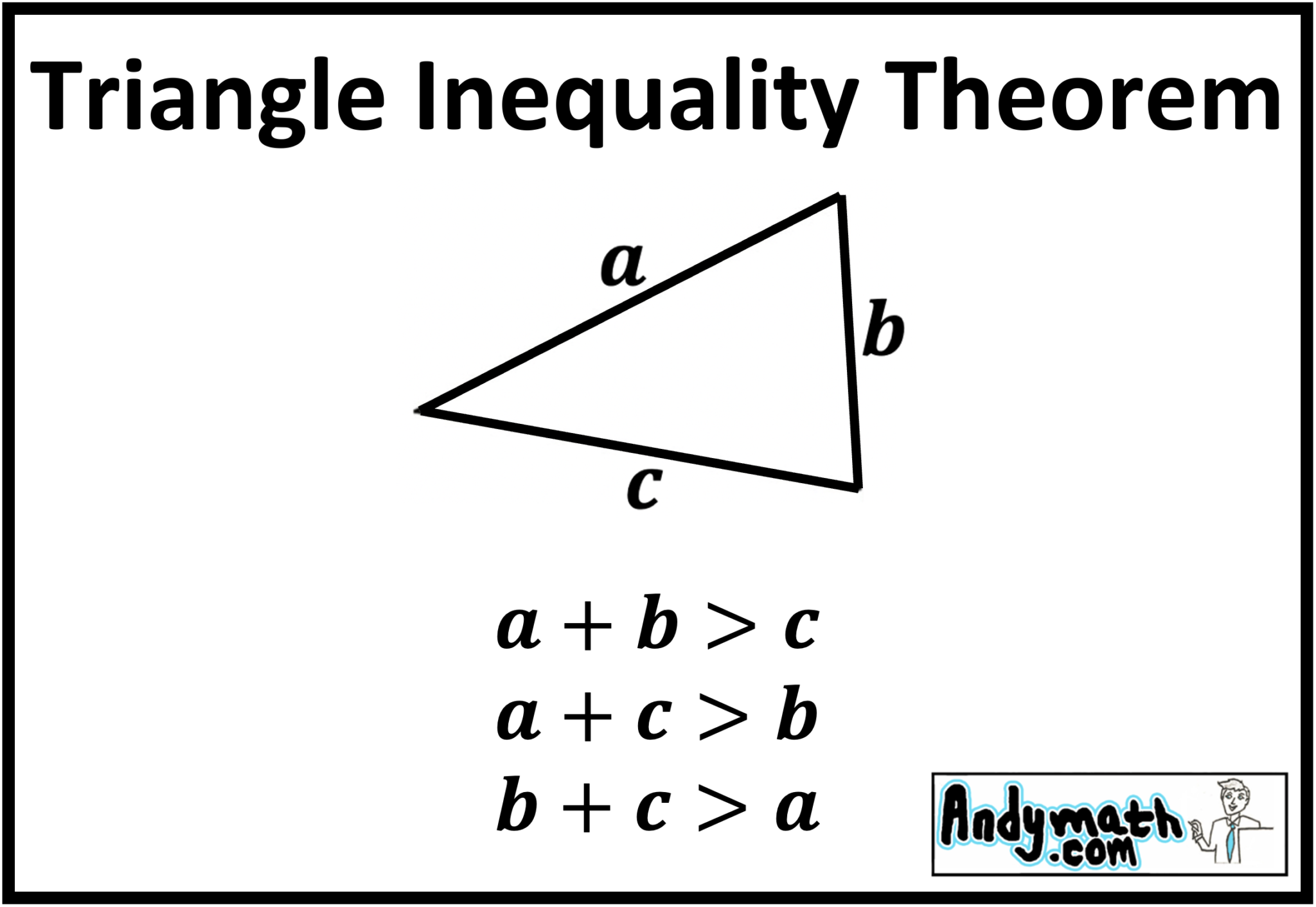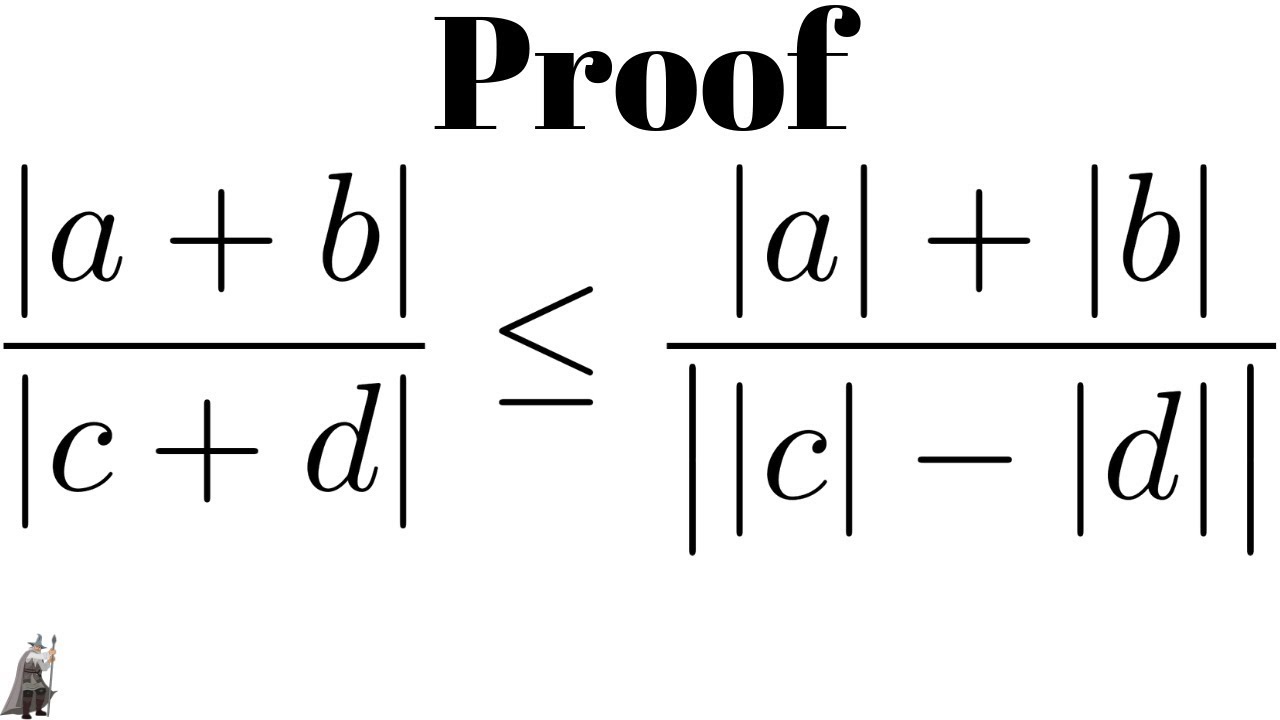1 That a metric must obey the triangle inequality is indeed one of the axioms of a metric space. - user1236 Jul 28, 2015 at 1:04 1 Consider the possibilities for a and b: each can be negative, zero, or positive. Thus there are at most nine possibilities to check out separately. You can do it! Be brave! - richard1941 Jan 24, 2018 at 1:18 2 The triangle inequality is a defining property of norms and measures of distance. This property must be established as a theorem for any function proposed for such purposes for each particular space: for example, spaces such as the real numbers, Euclidean spaces, the L p spaces ( p ≥ 1 ), and inner product spaces . Euclidean geometry
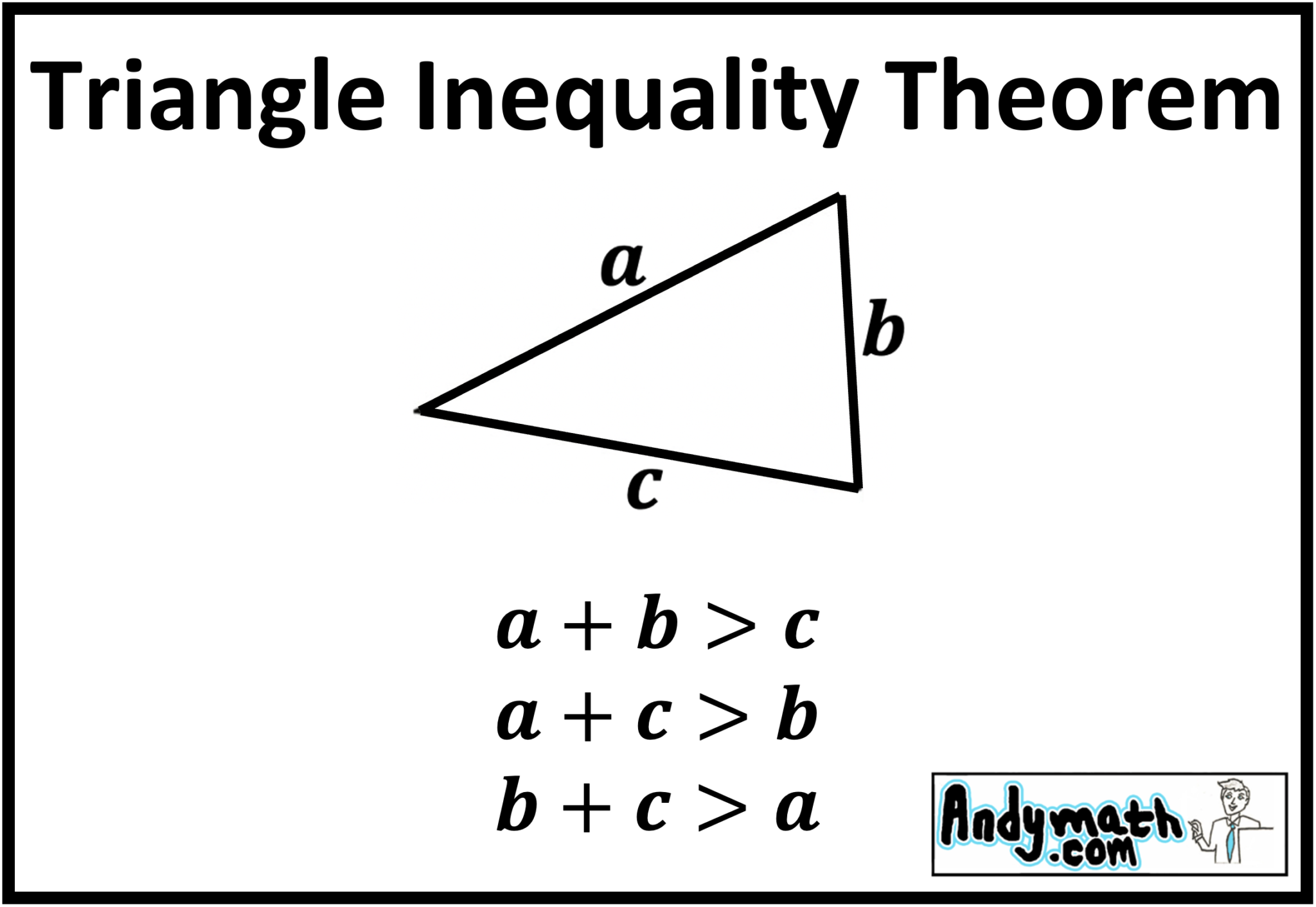
Triangle Inequality Theorem
To prove the theorem, assume there is a triangle ABC in which side AB is produced to D and CD is joined. Notice that the side BA of Δ ABC has been produced to a point D such that AD = AC. Now, since ∠BCD > ∠BDC. By the properties mentioned above, we can conclude that BD > BC. We know that, BD = BA + AD So, BA + AD > BC = BA + AC > BC The triangle inequality states that the sum of the lengths of any two sides of a triangle is greater than the length of the remaining side. It follows from the fact that a straight line is the shortest path between two points. The inequality is strict if the triangle is non- degenerate (meaning it has a non-zero area). Contents Examples Vectors The Cauchy-Schwarz Inequality holds for any inner Product, so the triangle inequality holds irrespective of how you define the norm of the vector to be, i.e., the way you define scalar product in that vector space. The triangle inequality theorem states that, in a triangle, the sum of lengths of any two sides is greater than the length of the third side. Suppose a, b and c are the lengths of the sides of a triangle, then, the sum of lengths of a and b is greater than the length c. Similarly, b + c > a, and a+ c > b.

How to Prove the Triangle Inequality for Complex Numbers YouTube
Proof: Extend BA to point D such that AD = AC, and join C to D, as shown below: We note that ∠ACD = ∠D, which means that in ∆ BCD, ∠BCD > ∠D. Sides opposite larger angles are larger, and thus: BD > BC AB + AD > BC AB + AC > BC (because AD = AC) This completes our proof. We can additionally conclude that in a triangle: Proof of triangle inequality theorem Thus, we can conclude that the sum of two sides of a triangle is greater than the third side. Video Lesson on BPT and Similar Triangles 2,36,417 Also, read: Triangles Isosceles Triangle Theorems Congruence Of Triangles Class 9 Triangles For Class 10 Example Problems Q.1. Triangle inequality theorem. The Triangle Inequality Theorem states that the sum of the lengths of any two sides of a triangle is greater than the length of the third side. Consider our 3−4−5 triangle example above. Add up any two sides of it. The sum of any of those two sides must be greater than the remaining side: 3+4>5 3 + 4 > 5. The biggest angle that a triangle can have is less than 180 degrees because the sum of the angle measures of a triangle is 180. Proof:. Now the whole principle that we're working on right over here is called the triangle inequality theorem and it's a pretty basic idea. That any one side of a triangle has to be less, if you don't want a.
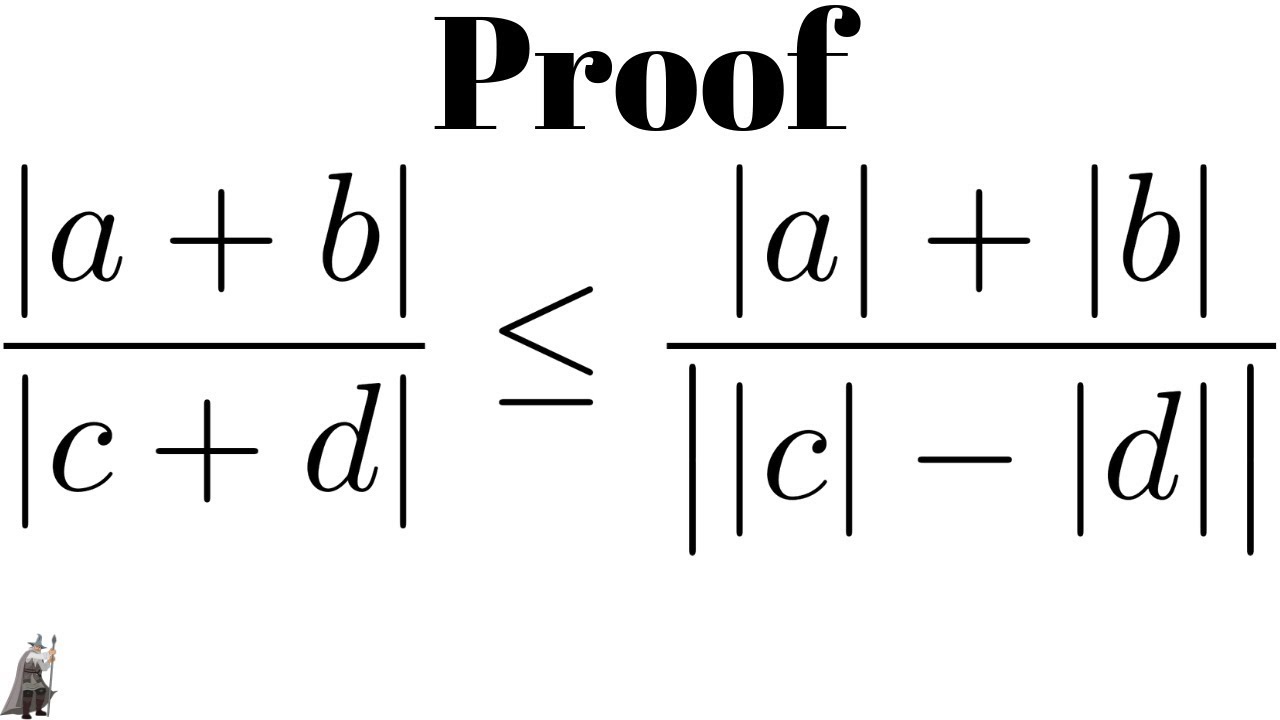
Inequality Proof using Both the Triangle Inequality and Reverse Triangle Inequality YouTube
The triangle inequality is a very simple inequality that turns out to be extremely useful. It relates the absolute value of the sum of numbers to the absolute values of those numbers. So before we state it, we should formalise the absolute value function. 🔗 Definition 5.4.1. Let , x ∈ R, then the absolute value of x is denoted | x | and is given by The absolute value of a sum is less than or equal to the sum of the absolute values for any two real numbers. That is: |a+b| is less than or equal to |a|+|b|.
Triangle Inequality. Let and be vectors. Then the triangle inequality is given by. (1) Equivalently, for complex numbers and , (2) Geometrically, the right-hand part of the triangle inequality states that the sum of the lengths of any two sides of a triangle is greater than the length of the remaining side. A generalization is. known that we can prove the triangle inequality in the broad sence, i.e. the ` '-version of (1.1), by algebraic argument. It is not exactly the triangle inequality in the sense of Euclidean geometry, because the point A is on the segment BC in case AB + AC = BC. For details of them, Subsection 1.2 (in particular Remark 1.3) will mention.

proof of CauchySchwarz inequality, proof of Triangle Inequality Calculus Coaches
Let us take our initial example. We could make a triangle with line segments having lengths 6, 8, and 10 units. This is because those line segments satisfy the triangle inequality theorem. 6 + 8 = 14 and 10 < 14. 8 + 10 = 18 and 6 < 18. 6 + 10 = 16 and 8 < 16. Use the Triangle Inequality Theorem. Check to make sure that the smaller two numbers add up to be greater than the largest number. 4 + 8 = 12 4 + 8 = 12 and 12 > 11 12 > 11 so yes these lengths make a triangle. Example 4.26.4 4.26. 4. Find the length of the third side of a triangle if the other two sides are 10 and 6.