Consider the line given by (4.6.2) (4.6.2). You can solve for the parameter t t to write. t = x − 1 t = y−2 2 t = z t = x − 1 t = y − 2 2 t = z. Therefore, x − 1 = y − 2 2 = z x − 1 = y − 2 2 = z. This is the symmetric form of the line. In the following example, we look at how to take the equation of a line from symmetric form. The parametric equations limit \(x\) to values in \((0,1]\), thus to produce the same graph we should limit the domain of \(y=1-x\) to the same. The graphs of these functions is given in Figure 9.25. The portion of the graph defined by the parametric equations is given in a thick line; the graph defined by \(y=1-x\) with unrestricted domain is.
Find Parametric Equations and Symmetric Equations for the Line
Now recall that in the parametric form of the line the numbers multiplied by \(t\) are the components of the vector that is parallel to the line. Therefore, the vector, \[\vec v = \left\langle {3,12, - 1} \right\rangle \] is parallel to the given line and so must also be parallel to the new line. The equation of new line is then, When parametrizing linear equations, we can begin by letting x = f ( t) and rewrite y wit h this parametrization: y = g ( t). Remember that the standard form of a linear equation is y = m x + b, so if we parametrize x to be equal to t, we'll have the following resulting parametric forms: x = f ( t) y = g ( t) x = t y = m t + b Answer The parametric form of the equation of a line passing through the point ( 𝑥, 𝑦) and parallel to the direction vector ( 𝑎, 𝑏) is 𝑥 = 𝑥 + 𝑎 𝑘, 𝑦 = 𝑦 + 𝑏 𝑘. We are given that our line has a direction vector ⃑ 𝑢 = ( 2, − 5) and passes through the point 𝑁 ( 3, 4), so we have ( 𝑥, 𝑦) = ( 3, 4), ( 𝑎, 𝑏) = ( 2, − 5). My Vectors course: https://www.kristakingmath.com/vectors-courseLearn how to find the parametric equations and symmetric equations of the line. GET EX.

Parametric Equations of Line Passing Through a Point YouTube
This is known as a parametric equation for the curve that is traced out by varying the values of the parameter t. t. Show that the parametric equation x=\cos t x = cost and y=\sin t y = sint (0 \leqslant t\leqslant 2\pi) (0 ⩽ t⩽ 2π) traces out a circle. Eliminating t t gives x^2+y^2= \cos^2 t+\sin^2 t=1, x2 +y2 = cos2 t+sin2 t = 1, Lesson 1: Vectors Vector intro for linear algebra Real coordinate spaces Adding vectors algebraically & graphically Multiplying a vector by a scalar Vector examples Scalar multiplication Unit vectors intro Unit vectors Add vectors Add vectors: magnitude & direction to component Parametric representations of lines Math > Linear algebra > d = ‖− − ⇀ aPM × ⇀ v‖ ‖ ⇀ v‖. Example 11.5.3: Calculating the Distance from a Point to a Line. Find the distance between the point M = (1, 1, 3) and line x − 3 4 = y + 1 2 = z − 3. Solution: From the symmetric equations of the line, we know that vector ⇀ v = 4, 2, 1 is a direction vector for the line. About Transcript In this video, we learn about parametric equations using the example of a car driving off a cliff. Parametric equations define x and y as functions of a third parameter, t (time). They help us find the path, direction, and position of an object at any given time. Created by Sal Khan. Questions Tips & Thanks
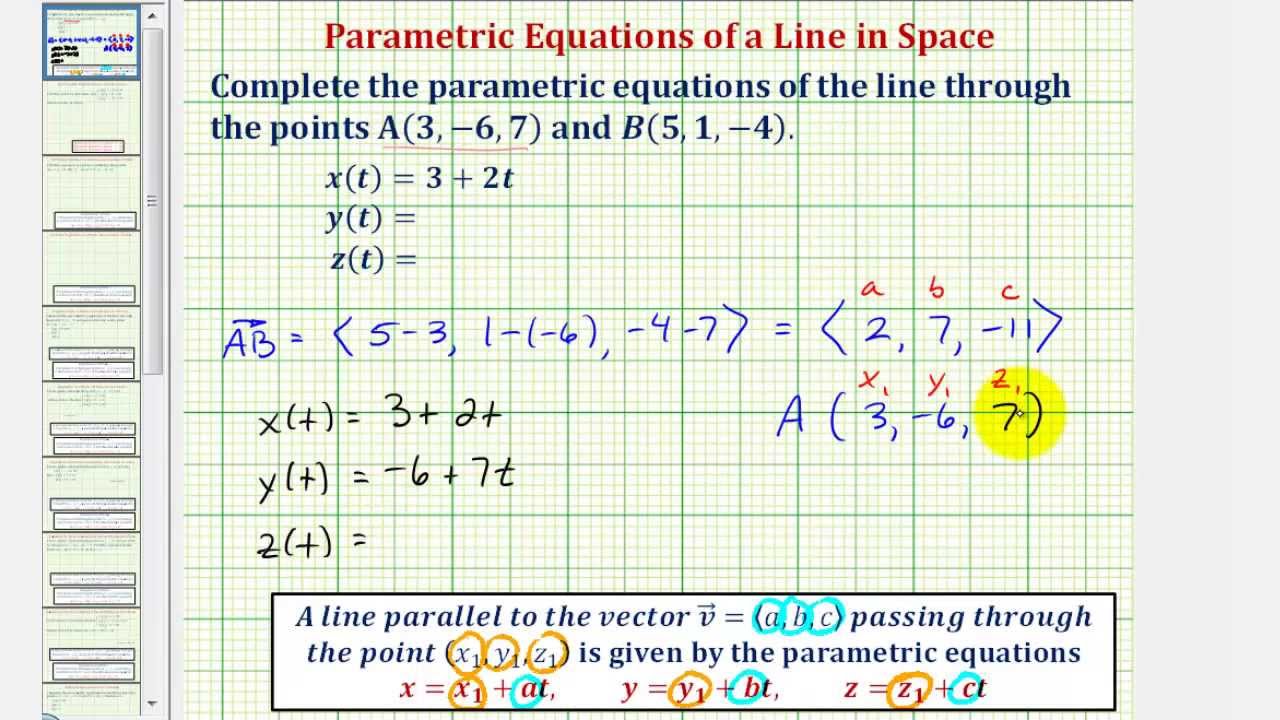
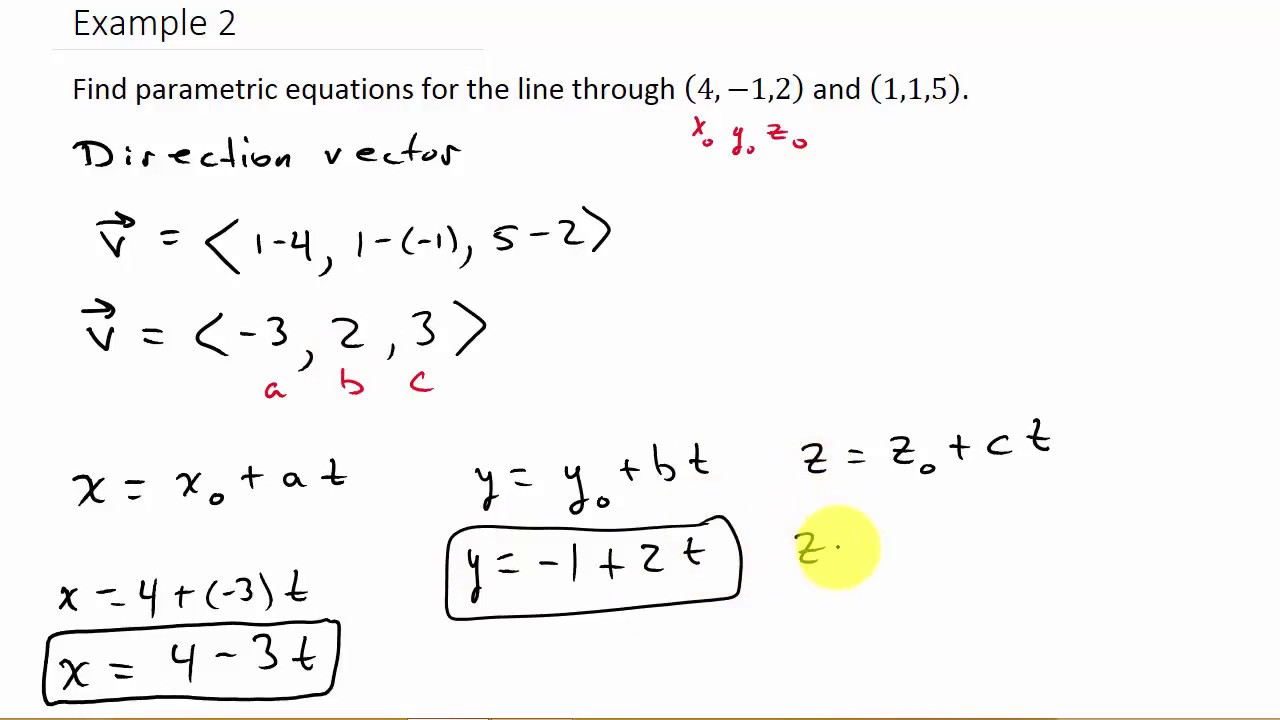
Ex Find the Parametric Equations of a Line in Space Given Two Points
−1 y(−1) =(−1)2 − 1 = 0 0 0 y(0) =(0)2 − 1 = −1 1 1 y(1) =(1)2 − 1 = 0 2 2 y(2) =(2)2 − 1 = 3 3 3 y(3) =(3)2 − 1 = 8 4 4 y(4) =(4)2 − 1 = 15 t y(t) = t2 − 1 y = x2 − 1 x(t) = t I know that to get the parametric equations of a line, you need a vector parallel to that line and a point on the line. So question 1) seems pretty straightforward. The vector PQ→ =< 2, −1, 3 > P Q → =< 2, − 1, 3 > is obviously parallel to the line since it includes the line. So the answer is x = 1 + 2t x = 1 + 2 t y = 2 − t y = 2 − t
Equations such as . are called parametric equations, and is called a parameter.. When given an equation of the form , we recognize it as an equation whose graph is a line and we don't need to make a table of values to sketch the graph of the equation.We should be able to do the same for parametric equations of lines. In the next Exploration we will examine our equations carefully to see if. In mathematics, a parametric equation defines a group of quantities as functions of one or more independent variables called parameters. [1] Parametric equations are commonly used to express the coordinates of the points that make up a geometric object such as a curve or surface, called a parametric curve and parametric surface, respectively.

How To Find The Vector Equation of a Line and Symmetric & Parametric
The collection of points that we get by letting t t be all possible values is the graph of the parametric equations and is called the parametric curve. To help visualize just what a parametric curve is pretend that we have a big tank of water that is in constant motion and we drop a ping pong ball into the tank. This Calculus 3 video tutorial explains how to find the vector equation of a line as well as the parametric equations and symmetric equations of that line in.




