In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares on the other two sides. Given two sides If you know two other sides of the right triangle, it's the easiest option; all you need to do is apply the Pythagorean theorem: a² + b² = c² If leg a is the missing side, then transform the equation to the form where a is on one side and take a square root: a = √ (c² - b²) If leg b is unknown, then: b = √ (c² - a²)
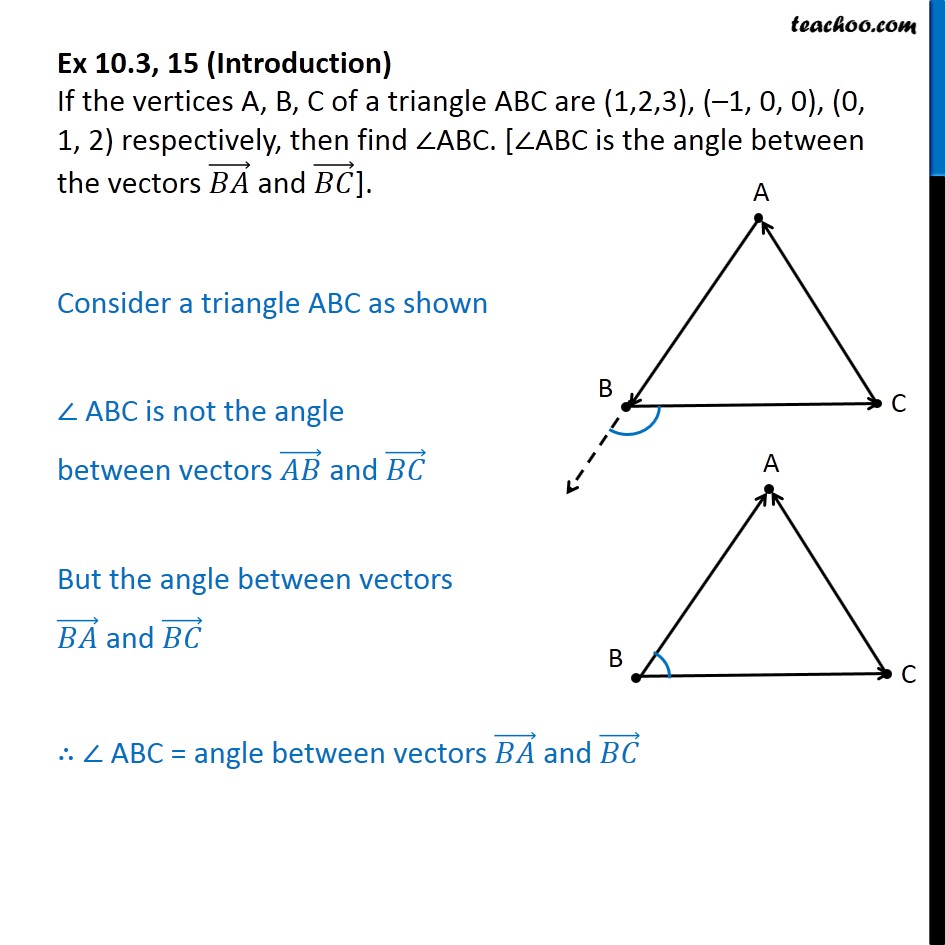
Ex 10.3, 15 If vertices A, B, C of triangle ABC are (1, 2, 3)
A=25 C=80 b=22 A=35 C=26 a=10 a=3 C=90 c=5. how to enter right-angled triangle. a=3 β=25 γ=45. triangle calc if we know the side and two angles. a=3 β=25 T=12. triangle calc, if know side, angle, and area of a triangle. T=2.5 c=2 b=4. find side a if we know sides b, c, and the area of triangle T. Naming angles and vertices Referencing the above triangles, an interior angle is formed at each vertex of a triangle. These angles share the same name as their vertices. Thus, the three interior angles for ABC above are A, B, and C. Triangle sides, angles, and congruence Perimeter of Triangle formula = a + b + c Area of a Triangle C M E ― Why are these words important? We're about to learn the trigonometric functions—sine, cosine, and tangent—which are defined using the words hypotenuse, opposite, and adjacent.

Example 6 In an isosceles triangle ABC with AB = AC Examples
C B A We are given the measure of angle ∠ B and the length of the hypotenuse , and we are asked to find the side opposite to ∠ B . The trigonometric ratio that contains both of those sides is the sine: sin ( ∠ B) = A C A B sin ( 40 ∘) = A C 7 ∠ B = 40 ∘, A B = 7 7 ⋅ sin ( 40 ∘) = A C Now we evaluate using the calculator and round: Where a and b are two sides of a triangle, and c is the hypotenuse, the Pythagorean theorem can be written as: a 2 + b 2 = c 2. EX: Given a = 3, c = 5, find b: 3 2 + b 2 = 5 2 9 + b 2 = 25 b 2 = 16 b = 4. Law of sines: the ratio of the length of a side of a triangle to the sine of its opposite angle is constant. Using the law of sines makes it. Calculator Use A right triangle is a special case of a triangle where 1 angle is equal to 90 degrees. In the case of a right triangle a 2 + b 2 = c 2. This formula is known as the Pythagorean Theorem. In our calculations for a right triangle we only consider 2 known sides to calculate the other 7 unknowns. Angles Add to 180°: A + B + C = 180°. When you know two angles you can find the third. 2. Law of Sines (the Sine Rule): a sin (A) = b sin (B) = c sin (C) When there is an angle opposite a side, this equation comes to the rescue. Note: angle A is opposite side a, B is opposite b, and C is opposite c. 3.

A triangle ABC with vertices A( 1,0), B( 2,3/4), and C( 1,2) has its orthocentre H . Then
Step 1: Enter the values of any two angles and any one side of a triangle below which you want to solve for remaining angle and sides. Triangle calculator finds the values of remaining sides and angles by using Sine Law. Sine law states that a sinA = b sinB = c sinC a sin A = b sin B = c sin C Cosine law states that- sin (A) < a/c, there are two possible triangles. solve for the 2 possible values of the 3rd side b = c*cos (A) ± √ [ a 2 - c 2 sin 2 (A) ] [1] for each set of solutions, use The Law of Cosines to solve for each of the other two angles. present 2 full solutions. Example: sin (A) = a/c, there is one possible triangle.
The perimeter of a triangle is equal to the sum of all the sides of the triangle, and the formula is expressed as, Perimeter of a triangle formula, P = (a + b + c), where 'a', 'b', and 'c' are the three sides of the triangle. The equilateral triangle formula for perimeter is, Perimeter of equilateral triangle = (a +a + a) = 3a. For similar triangles A B C and X Y Z shown below: X Y = k ( A B) Y Z = k ( B C) X Z = k ( A C) X Y A B = Y Z B C = X Z A C = k. A B C X Y Z. To calculate a missing side length, we: Write a proportional relationship using two pairs of corresponding sides. Plug in known side lengths. We need to know 3.
"Triangle B, No. 1" by Walter Stomps, Jr Caza Sikes Art Fine Art Appraisers
The Law of Sines. The Law of Sines (or Sine Rule) is very useful for solving triangles: a sin A = b sin B = c sin C. It works for any triangle: a, b and c are sides. A, B and C are angles. (Side a faces angle A, side b faces angle B and. side c faces angle C). the third side of a triangle when we know two sides and the angle between them (like the example above) the angles of a triangle when we know all three sides (as in the following. = a 2 + b 2 − c 2 2ab. cos(A) = b 2 + c 2 − a 2 2bc. cos(B) = c 2 + a 2 − b 2 2ca. Example: Find Angle "C" Using The Law of Cosines (angle version) In this.




