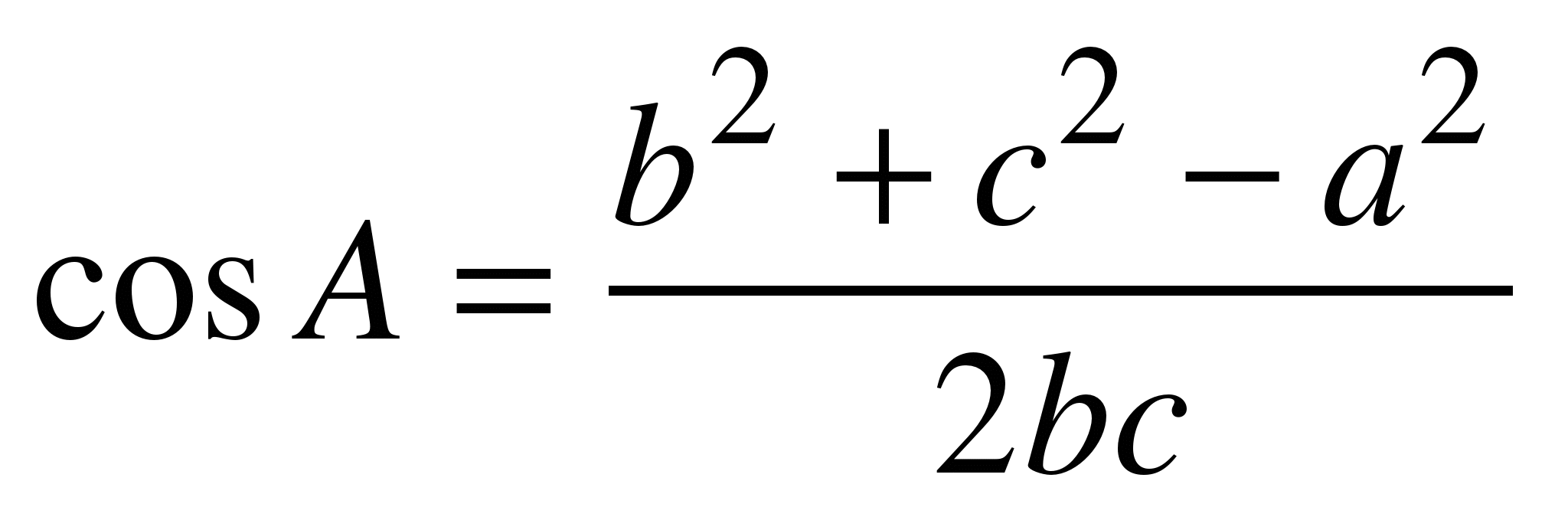In trigonometry, cos (a - b) is one of the important trigonometric identities, that finds application in finding the value of the cosine trigonometric function for the difference of angles. The expansion of cos (a - b) helps in representing the cos of a compound angle in terms of trigonometric functions sine and cosine. Cosines Tangents Cotangents Pythagorean theorem Calculus Trigonometric substitution Integrals ( inverse functions) Derivatives v t e In trigonometry, trigonometric identities are equalities that involve trigonometric functions and are true for every value of the occurring variables for which both sides of the equality are defined.
Trigonometry
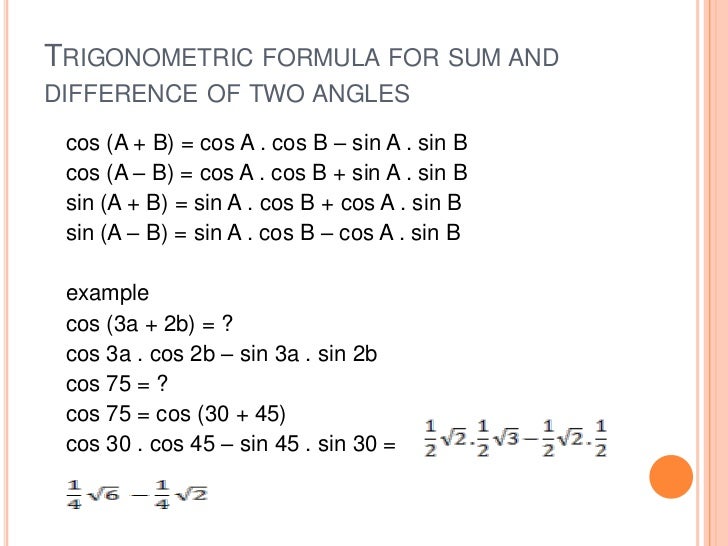
Maths Math Formula Trigonometry Formulas Trigonometry Formulas In Trigonometry, different types of problems can be solved using trigonometry formulas. These problems may include trigonometric ratios (sin, cos, tan, sec, cosec and cot), Pythagorean identities, product identities, etc. Formula ( 1). cos ( a + b) = cos a cos b − sin a sin b ( 2). cos ( x + y) = cos x cos y − sin x sin y Introduction Let us consider that a and b are two variables, which denote two angles. The sum of two angles is written as a + b, which is actually a compound angle. The Law of Cosines (also called the Cosine Rule) says: c 2 = a 2 + b 2 − 2ab cos (C) It helps us solve some triangles. Let's see how to use it. Example: How long is side "c". ? We know angle C = 37º, and sides a = 8 and b = 11 The Law of Cosines says: c2 = a2 + b2 − 2ab cos (C) Put in the values we know: c2 = 82 + 112 − 2 × 8 × 11 × cos (37º) Because of all that we can say: sin (θ) = 1/csc (θ) cos (θ) = 1/sec (θ) tan (θ) = 1/cot (θ) And the other way around: csc (θ) = 1/sin (θ) sec (θ) = 1/cos (θ) cot (θ) = 1/tan (θ) And we also have: cot (θ) = cos (θ)/sin (θ) Pythagoras Theorem

Understanding Cos A+B Formula
19 I know that there is a trig identity for cos ( a + b) and an identity for cos ( 2 a), but is there an identity for cos ( a b)? cos ( a + b) = cos a cos b − sin a sin b cos ( 2 a) = cos 2 a − sin 2 a cos ( a b) =? trigonometry Share Cite asked May 8, 2014 at 22:36 TechMaster100 499 2 6 13 2 In this explainer, we will learn how to use Euler's formula to prove trigonometric identities like cos(A+B)= cosA.cosB- sinA.sinBand sin(A+B)= sinA.cosB+ sin. The trigonometric identity Cos A - Cos B is used to represent the difference of cosine of angles A and B, Cos A - Cos B in the product form using the compound angles (A + B) and (A - B). We will study the Cos A - Cos B formula in detail in the following sections. Cos A - Cos B Difference to Product Formula Trigonometric Identities Purplemath What is an identity? In mathematics, an "identity" is an equation which is always true, regardless of the specific value of a given variable. An identity can be "trivially" true, such as the equation x = x or an identity can be usefully true, such as the Pythagorean Theorem's a2 + b2 = c2 MathHelp.com
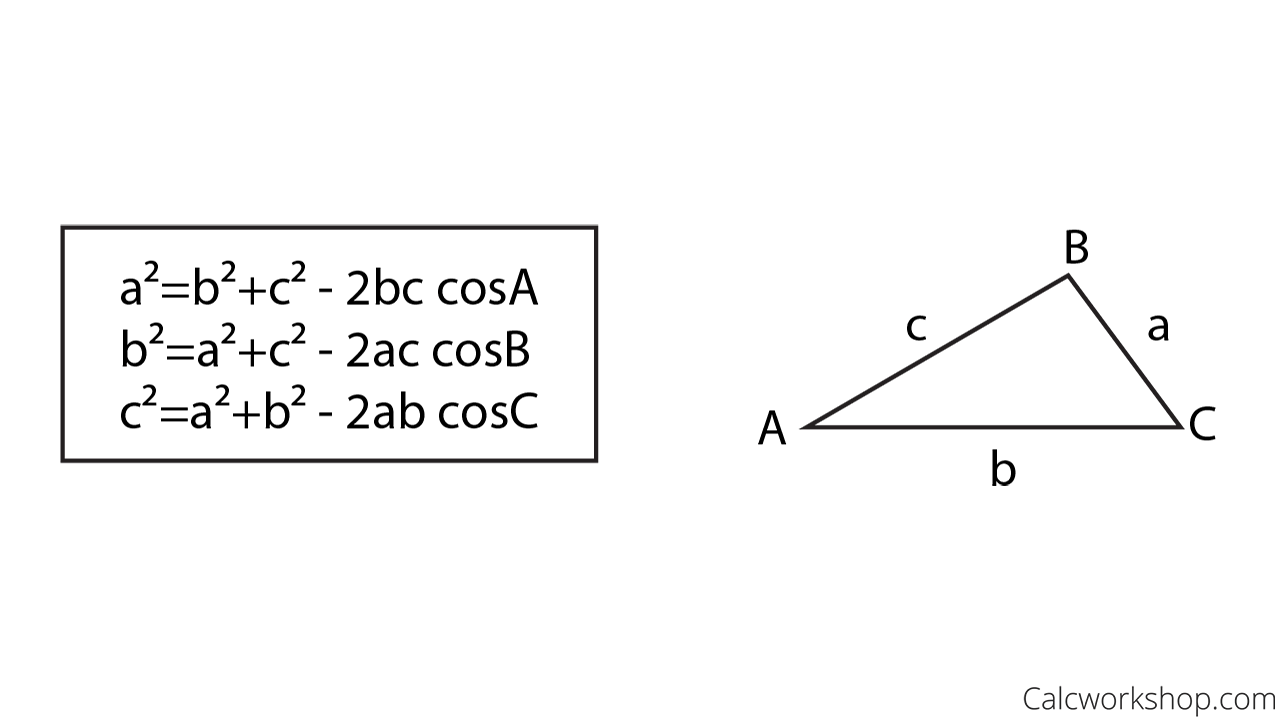
What is the Law of Cosines? (Explained in 3 Powerful Examples!)
Get Started Cos (a + b) In trigonometry, cos (a + b) is one of the important trigonometric identities involving compound angle. It is one of the trigonometry formulas and is used to find the value of the cosine trigonometric function for the sum of angles. cos (a + b) is equal to cos a cos b - sin a sin b. The formula of cos (a+b)cos (a-b) is given by cos (a+b)cos (a-b) = cos 2 a -sin 2 b. In this post, we will establish the formula of cos (a+b) cos (a-b). Note that cos (a+b) cos (a-b) is a product of two cosine functions. We will use the following two formulas: cos (a+b) = cos a cos b - sin a sin b. (i) cos (a-b) = cos a cos b + sin a sin b. (ii)
cos^2(a + b) = cos^2(a) + cos^2(b) - 2cos(a)cos(b). Proof of Cos(a + b) Formula. The cos(a+b) formula is a mathematical expression used to determine the angle of two vectors. The formula is derived from the law of cosines, which states that the cosine of the angle between two vectors is equal to the product of their magnitudes and the sum of. The formula of cos (A + B) is cos A cos B - sin A sin B. Example : If sin A = 3 5 and cos B = 9 41, find the value of cos (A + B). Solution : We have, sin A = 3 5 and cos B = 9 41 ∴ cos A = 1 - s i n 2 A and sin B = 1 - c o s 2 B cos A = 1 - 9 25 = 4 5 and sin B = 1 - 81 1681 = 40 41 Now, By using above formula,
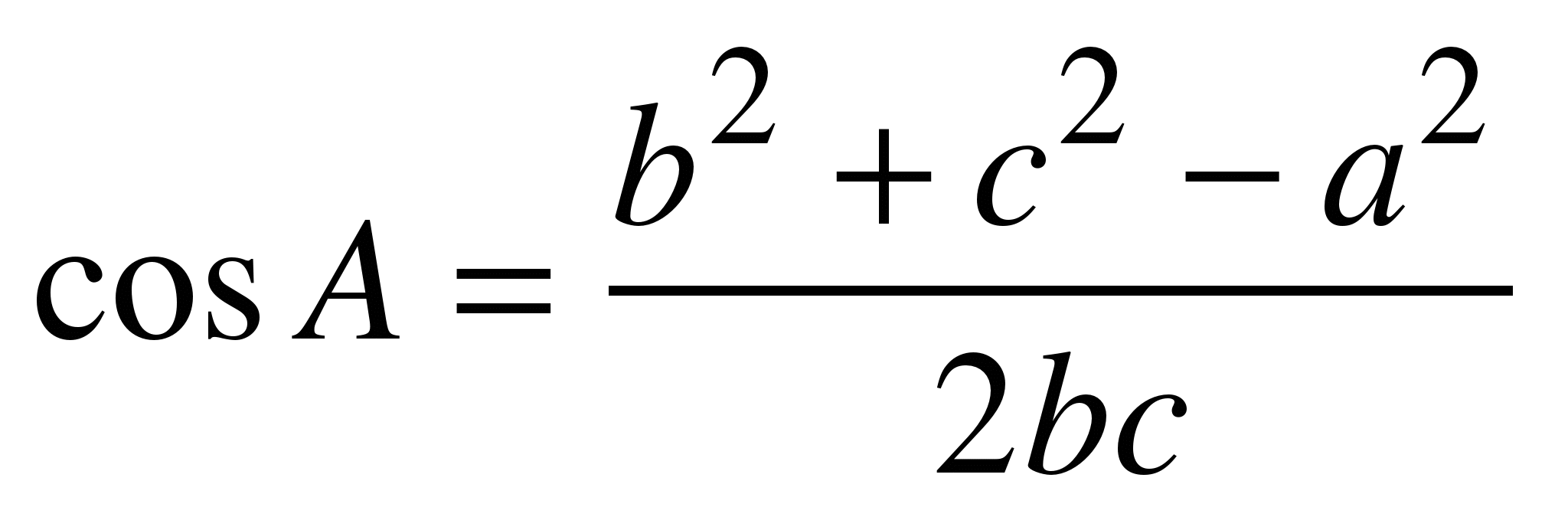
the Cosine Rule National 5 Maths
Learn to derive the formula of cos (A + B). Proof of expansion of cos(A+B). cos (A +B) is an important trigonometric identity. We all learn the expansion and. Cos a Cos b is a trigonometric formula that is used in trigonometry. Cos a cos b formula is given by, cos a cos b = (1/2) [cos (a + b) + cos (a - b)].