Steps for Completing the square method. Suppose ax2 + bx + c = 0 is the given quadratic equation. Then follow the given steps to solve it by completing the square method. Step 1: Write the equation in the form, such that c is on the right side. Step 2: If a is not equal to 1, divide the complete equation by a such that the coefficient of x2. Completing the Square Method Class 10 In this section, you will learn how to solve a quadratic equation by completing the square method.. In the given quadratic equation ax + bx + c = 0, divide the complete equation by a (coefficient of x ). If the coefficient of x is 1 (a = 1), the above process is not required.

Completing the Square Calculator Method, Explanation and Problems Still Education
Say you have the equation 3x^2-6x+8=23. To complete the square, first, you want to get the constant (c) on one side of the equation, and the variable (s) on the other side. To do this, you will subtract 8 from both sides to get 3x^2-6x=15. Next, you want to get rid of the coefficient before x^2 (a) because it won´t always be a perfect square. 📚Algebra Handwritten Notes link: https://drive.google.com/file/d/1cZNsWiBG6HFzubjo5TjUHdoQ4nwFw5TI/view?usp=sharing📚Geometry Handwritten Notes link: https:. Exam Special Series Playlist: https://youtube.com/playlist?list=PLK4eRC6zQUX6ZXeTew9-8qh6QwLYVuShF🔥Study Materials🔥📚Algebra Handwritten Notes link: https:. Step 1: Write the quadratic equation as x 2 + bx + c. ( Coefficient of x 2 needs to be 1. If not, take it as the common factor.) Step 2: Determine half of the coefficient of x. Step 3: Take the square of the number obtained in step 1.
How To Complete Square Completing the Square Examples MathBitsNotebook(A1 CCSS Math) The
We can use a method called completing the square. Let's start with the solution and then review it more closely. ( 1) x 2 + 6 x = − 2 ( 2) x 2 + 6 x + 9 = 7 Add 9, completing the square. ( 3) ( x + 3) 2 = 7 Factor the expression on the left. ( 4) ( x + 3) 2 = ± 7 Take the square root. ( 5) x + 3 = ± 7 ( 6) x = ± 7 − 3 Subtract 3. This algebra 2 video tutorial shows you how to complete the square to solve quadratic equations. This is for high school students taking algebra and univers. Rewrite the equation by completing the square. 2 x 2 − 9 x + 7 = 0. ( x + ) 2 =. Show Calculator. Stuck? Review related articles/videos or use a hint. Report a problem. Do 4 problems. Learn for free about math, art, computer programming, economics, physics, chemistry, biology, medicine, finance, history, and more. x 2 + 10 x = − 24. We complete the square by taking half of the coefficient of our x term, squaring it, and adding it to both sides of the equation. Since the coefficient of our x term is 10 , half of it would be 5 , and squaring it gives us 25 . x 2 + 10 x + 25 = − 24 + 25. We can now rewrite the left side of the equation as a squared term.

10th Class Math Chapter 1 exercise 1.1 Question 3 Lec 4 Completing square method YouTube
10: Quadratic Equations In this video i'll show how to solve any quadratic equation by Completing Square Method step by step. This video will b very helpful for students having issues regarding solution of quadratic.
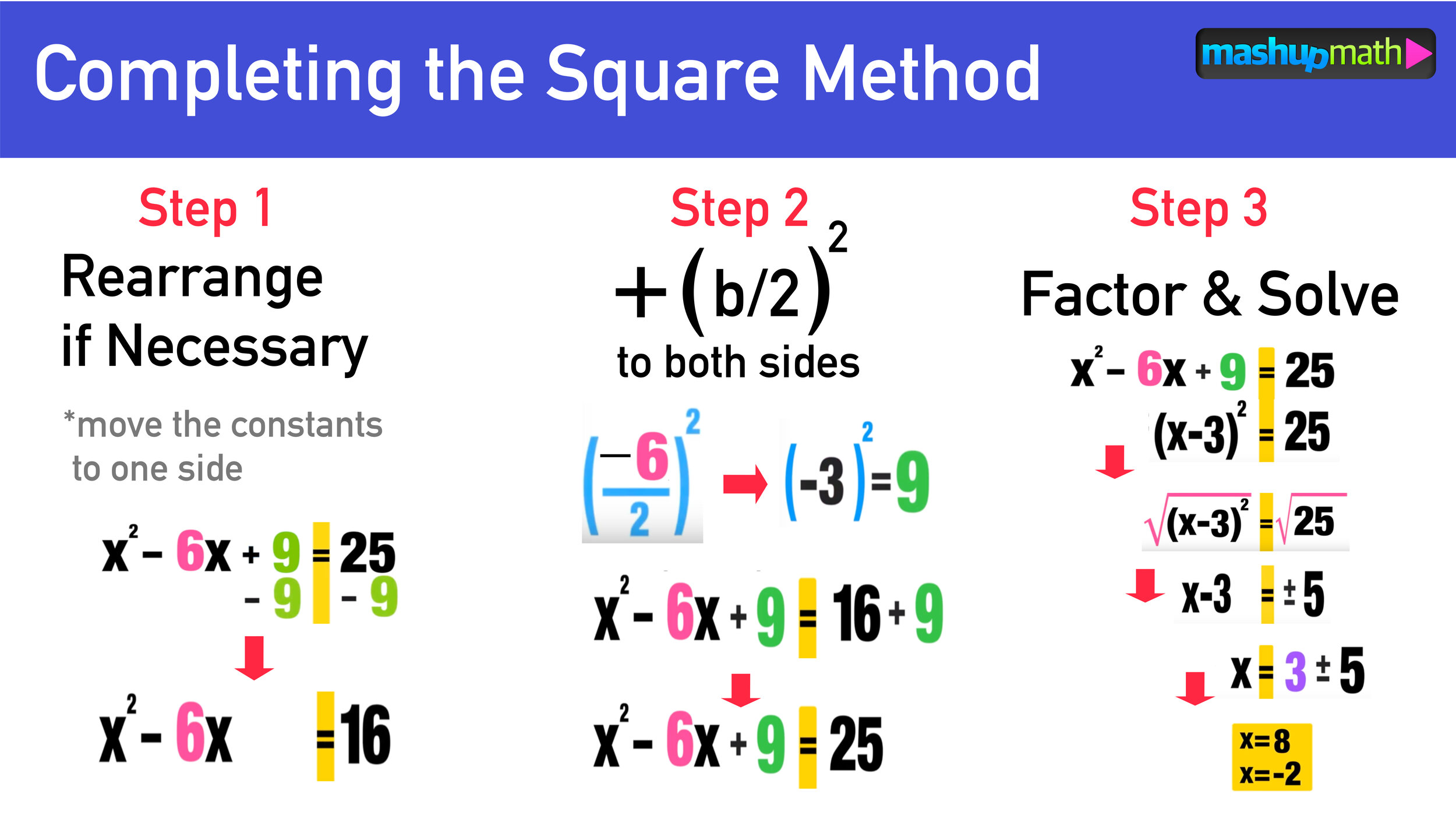
Completing the Square. In this section, we will devise a method for rewriting any quadratic equation of the form \[a x^{2}+b x+c=0\] in the form \[(x-p)^{2}=q\] This process is called completing the square. As we have seen, quadratic equations in this form can easily be solved by extracting roots. We begin by examining perfect square trinomials: Step 1 Divide all terms by a (the coefficient of x2 ). Step 2 Move the number term ( c/a) to the right side of the equation. Step 3 Complete the square on the left side of the equation and balance this by adding the same value to the right side of the equation. We now have something that looks like (x + p) 2 = q, which can be solved this way:

Class 10th Maths Lecture 6 Completing square method Part 2 Ch4th YouTube
Solution: Step 1: Eliminate the constant on the left side, and then divide the entire equation by [latex] - \,3 [/latex]. Step 2: Take the coefficient of the linear term which is [latex] {2 \over 3} [/latex]. Divide it by [latex]2 [/latex] and square it. Step 3: Add the value found in step #2 to both sides of the equation. Completing the square method for Exercise 4.3 Quadratic equations maths class 10 Subject Teacher 1.21M subscribers Join Subscribe 36K Share 1.4M views 6 years ago Chapter 4 Quadratic Equations.




