Integration Formulas www.mathportal.org Integration Formulas 1. Common Integrals Indefinite Integral Method of substitution ∫ f ( g ( x )) g ′ ( x ) dx = ∫ f ( u ) du Integration by parts ∫ f ( x ) g ′ ( x ) dx = f ( x ) g ( x ) − ∫ g ( x ) f ′ ( x ) dx Integrals of Rational and Irrational Functions + 1 ∫ x dx n xn = + C + 1 ∫ dx = ln x + C x ∫ Click here to download the PDF of important basic integral formulas. Download Integral Formulas PDF Also, check: Differentiation formulas Integration Rules

Integration formulas Handwritten Notes PDF Download
Differentiation Formulas d dx k = 0 (1) d dx [f(x)±g(x)] = f0(x)±g0(x) (2) d dx [k ·f(x)] = k ·f0(x) (3) d dx [f(x)g(x)] = f(x)g0(x)+g(x)f0(x) (4) d dx f(x) g(x. Symbolab Integrals Cheat Sheet Common Integrals: ∫𝑥−1 𝑥=ln(𝑥) ∫ 𝑥 𝑥 =ln(𝑥) ∫ |𝑥 𝑥=𝑥√𝑥 2 2 ∫ 𝑥 𝑥= 𝑥 ∫sin(𝑥) 𝑥=−cos(𝑥) functions at the bottom of the list are more like to be. dv. Trig Integrals: Integrals involving sin(x) and cos(x): Integrals involving sec(x) and tan(x): 1. If the power of the sine is odd and positive: Goal: Basic integration formulas 1. k dx = kx + C xn+1 2. xndx = + C n + 1 1 3. dx = ln |x| + C x 4. ex dx = ex + C 5. axdx ax = + C ln(a) 6. sin(x) dx = − cos(x) + C 7. cos(x) dx = sin(x) + C 8. sec2(x) dx = tan(x) + C 9. csc2(x) dx = − cot(x) + C 10. sec(x) tan(x) dx = sec(x) + C 11. csc(x) cot(x) dx = − csc(x) + C (any number k) (n 6= −1)

Physics Wood BASIC DIFFERENTIATION & INTEGRATION FORMULA
Integrals with Trigonometric Functions Z sinaxdx= 1 a cosax (63) Z sin2 axdx= x 2 sin2ax 4a (64) Z sinn axdx= 1 a cosax 2F 1 1 2; 1 n 2; 3 2;cos2 ax (65) Z sin3 axdx= 3cosax 4a + cos3ax 12a (66) Z cosaxdx= 5.4 Integration Formulas and the Net Change Theorem; 5.5 Substitution; 5.6 Integrals Involving Exponential and Logarithmic Functions;. If you are redistributing all or part of this book in a digital format, then you must include on every digital page view the following attribution: the integrals of specific functions and structural type formulas. Each formula for the derivative of a specific function corresponds to a formula for the derivative of an elementary function. The following table lists integration formulas side by side with the corresponding differentiation formulas. Z xn dx = xn+1 n+1 if n 6= −1 d dx (xn. Techniques of Integration Over the next few sections we examine some techniques that are frequently successful when seeking antiderivatives of functions. Sometimes this is a simple problem, since it will be apparent that the function you wish to integrate is a derivative in some straightforward way. For example, faced with Z x10 dx
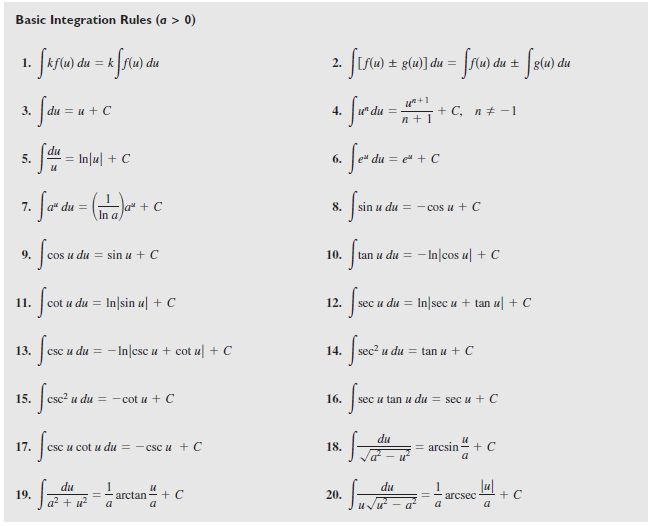
Basic Integration Rules A Freshman's Guide to Integration
heading of each section. And I will NOT write the integration constant +Cin the notes to save some ink, but you should always write it!! 2 Introduction to the Integral 2.1 (5.1) Area and Distances 2.1.1 Area A mathematical illustrative example of the integral is area under a curve. Let f(x) be a non-negative continuous function. Integration Formulas: (1) Z xp dx = xp+1 p+1 +C; p 6= 1 (2) Z sin(x)dx = cos(x)+C (3) Z cos(x)dx = sin(x)+C (4) Z sec2(x)dx = tan(x)+C (5) Z csc2(x)dx = cot(x)+C (6) Z sec(x)tan(x)dx = sec(x)+C (7) Z csc(x)cot(x)dx = csc(x)+C (8) Z 1 x dx = ln jx j+C (9) Z tan(x)dx = ln jcos(x) j+C = ln jsec(x) j+C (10) Z sec(x)dx = ln jsec(x)+tan(x) j+C
Guidelines for Integration by Substitution. 1. Let u be a function of x (usually part of the integrand). 2. Solve for x and dx in terms of u and du. 3. Convert the entire integral to u-variable form and try to fit it to one or more of the basic integration formulas. If none fits, try a different substitution. 1.5. Integration by Parts 21 1.6. Trigonometric Integrals and Trigonometric Substitutions 26 1.7. Partial Fractions 32 1.8. Integration using Tables and CAS 39 1.9. Numerical Integration 41 1.10. Improper Integrals 46 Chapter 2. Applications of Integration 50 2.1. More about Areas 50 2.2. Volumes 52 2.3. Arc Length, Parametric Curves 57 2.4.
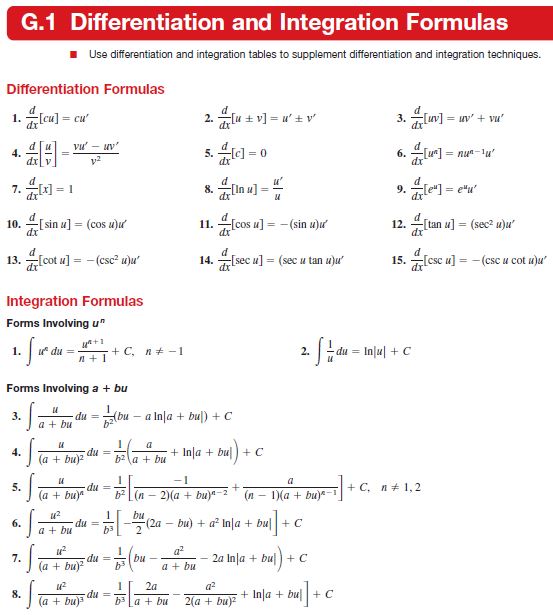
Integration Formulas List
INTEGRATION FORMULAE.pdf Basic Integration Formulas Integral of special functions Integral by Partial Fractions Integration by Parts Other Special Integrals Area as a sum Properties of definite integration Integration of Trigonometric Functions, Properties of Definite Integration are all mentioned here. Tired of ads? Get Ad-free version of Teachoo for ₹ 999 ₹499 per month




