The continuity of a function and the differentiability of a function are complementary to each other. The function y = f (x) needs to be first proved for its continuity at a point x = a, before it is proved for its differentiability at the point x = a. x^2 is a parabola centered at the origin..If you take its derivative you get 2x, therefore the derivative of f (x) at 0 would be equal to 0. or you can write as f' (0) = 0..It is a parabola you do not have a hard corner where you would end up with an infinite number of slopes crossing that point.. Comment ( 40 votes) Upvote Downvote Flag

Differentiation Formula Continuity and Differentiability Class12 Maths Part 6 Chapter 5
LearnPick does not verify the identity or authenticity of information posted by tutors or students. For more information on verifying the identity of information posted by other users, please visit our Safety Centre. Notes on Formula Sheet Of Chapter 5 Continuity & Differentiability Class 12 Maths compiled by Pawan Kumar. Continuity and differentiability are one of the most important topics which make the students understand some of the concepts such as continuity on an interval, continuity at a point, derivative of functions, and etc. 'f' is a real function that has point 'c' in its domain, then 'f' is said to be a continuous function if the value of the functio. More formally, we make the following definition. Definition 1.7. A function f f is continuous at x = a x = a provided that. (a) f f has a limit as x → a x → a, (b) f f is defined at x = a x = a, and. (c) limx→a f(x) = f(a). lim x → a f ( x) = f ( a). Conditions (a) and (b) are technically contained implicitly in (c), but we state them. Continuity and Differentiability is one of the most important topics which help students to understand the concepts like, continuity at a point, continuity on an interval, derivative of functions and many more. However, continuity and Differentiability of functional parameters are very difficult. Let us take an example to make this simpler:
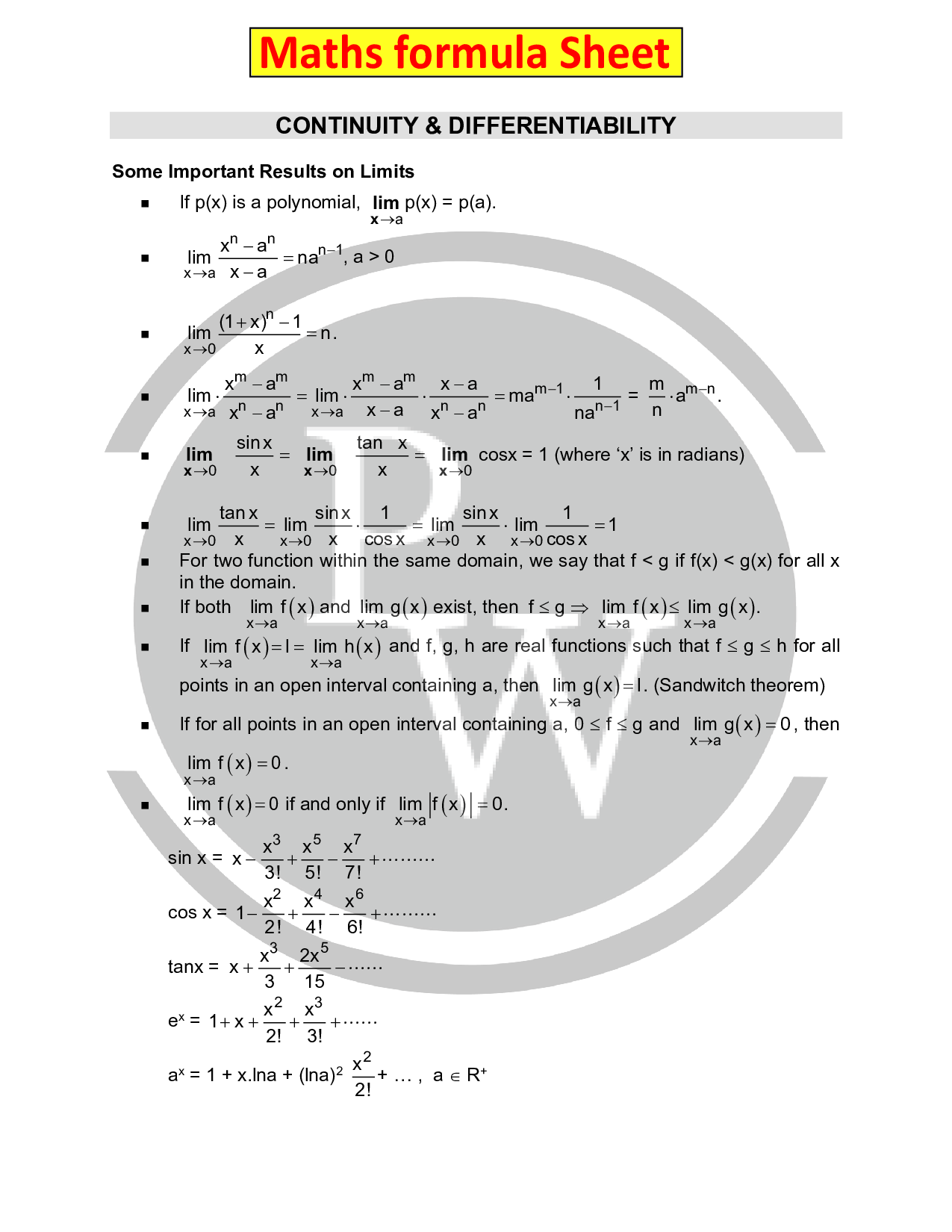
Chapter Continuity and differentiability Class 11 maths formula
It means that a function is differentiable everywhere its derivative is defined. So, as long as you can evaluate the derivative at every point on the curve, the function is differentiable. How To Determine Differentiability By using limits and continuity! The definition of differentiability is expressed as follows: About Transcript We examine a piecewise function to determine its continuity and differentiability at an edge point. By analyzing left and right hand limits, we establish continuity. Checking the limit of the difference quotient confirms both left and right hand limits are equal, making the function continuous and differentiable at the edge point. Quiz Unit test Continuity and differentiability Intuition for the definition of continuity Continuity of a function using only definition 1. In an open interval (a, b), a function f is said to be continuous if it is continuous at all points in the interval. 2. In an closed interval [a, b], a function f is said to be continuous if f is continuous in (a, b) , lim x → a + f(x) = f(a) , lim x → b − f(x) = f(b) .

PPT BCC.01.9 Continuity and Differentiability of Functions PowerPoint Presentation ID257105
CONTINUITY AND DIFFERENTIABILITY vThe whole of science is nothing more than a refinement of everyday thinking." — ALBERT EINSTEIN v 5.1 Introduction This chapter is essentially a continuation of our study of differentiation of functions in Class XI. Chapter 5 Class 12 Continuity and Differentiability; Concept wise; Finding derivative of a function by chain rule; Finding derivative of a function by chain rule.. Differentiation forms the basis of calculus, and we need its formulas to solve problems. We have prepared a list of all the Formulas Basic Differentiation Formulas
Mathematically, this is expressed as: f' (c) = lim [x → c] (f (c + h) - f (c)) / h. In simpler terms, a function is differentiable at a point if it has a well-defined tangent line at that point. The tangent line represents the instantaneous rate of change of the function. Also Check - solid shapes Formula Relationship: To understand the principles of continuity and differentiability, students should become familiar with the relevant mathematical formulas. Theorems on Continuity and Differentiability. Theorem 1: If two functions f(x) and g(x) are continuous at a real valued function and continuous at a point x = c, we have:

See complete solutions of Miscellaneous Exercise(Continuity & Differentiability) with PDF NCERT
"Continuity and Differentiability One Shot Video: https://youtu.be/3v--OCXUgYYTimestamp:00:00 Introduction00:53 Continuity03:17 Algebra of Continuity 04:01 C. Continuity and Differentiability is an important unit in class 12 mathematics from the perspective of both boards and other competitive exams. It provides in-depth knowledge about the basics of continuity, differentiability, and the relation between them.




