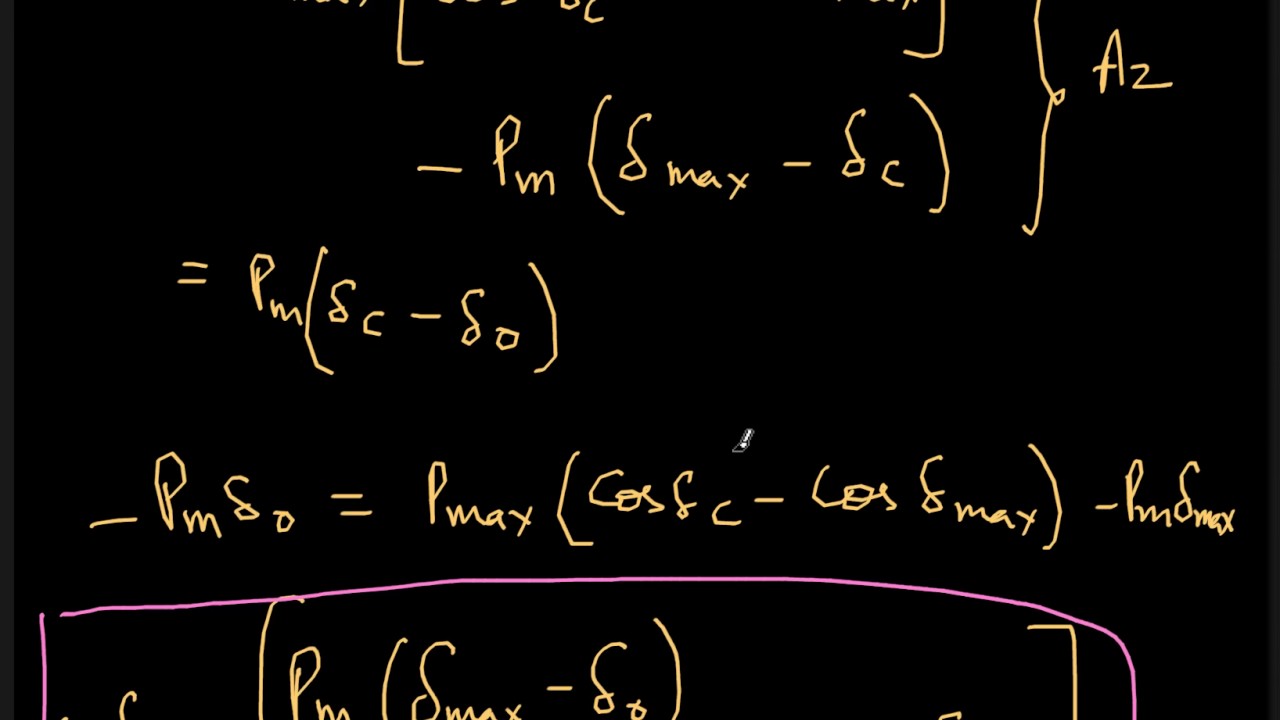The critical clearing angle is defined as the maximum change in the load angle curve before clearing the fault without loss of synchronism. In other words, when the fault occurs in the system the load angle curve begin to increase, and the system becomes unstable. The angle at which the fault becomes clear and the system becomes stable is called critical clearing angle. There is a critical angle within which the fault must be cleared if the system is to remain stable and the equal-area criterion is to be satisfied. This angle is known as the critical clearing angle. Consider the system of Fig. 11.9 operating with mechanical input Pi at stady angle δ0. (Pi=Pe) as shown by the point 'a' on the power angle.

Critical Clearing Angle and Critical Clearing Time YouTube
About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How YouTube works Test new features NFL Sunday Ticket Press Copyright. In the above video we derive critical clearing angle by using equal area criterion .. We take one system and we see how to find critical clearing angle for t. The first critical clearing angle δ c is denoted by δ c,1 and its corresponding CCT as t 1. If we do not clear the fault at δ = δ c, the rotor angle will increase and move forward to δ max (from left to right in figure 9a) and then move back (from right to left). Here, the clearing angle is given by δ cr, the critical clearing angle. Since, A 2 = A 1. We get The critical clearing angle is related to the equality of areas, it is termed as equal area criterion. It can be used to find out the utmost limit on the load which the system can acquire without crossing the stability limit.

Curve of The critical clearing angle cr about 47.8529 is placed... Download Scientific Diagram
This video series is based on power system Stability covers all terminology of Power System Stability- its types , techniques , controlling & Synchronizing w. -The fault clearing time is assumed longer than the critical clearing time of the three-phase fault - unstable system in case of 3-phase fault (since area 1-2-3-4 > area 4-5-8). -For the 1-phase fault, the accelerating torque is smaller, thus the rotor will accelerate less rapidly. By the time the fault is cleared, the rotor reaches point 10. The critical conditions. such as critical clearing time (CCT) and critical clearing angle (CCA) were obtained. The computation of CCA and CCT is curried out. step by step using the characteristics of the faulted and postfault trajectories from given initial conditions until their intersection. point. The critical conditions such as critical clearing time (CCT) and critical clearing angle (CCA) were obtained. The computation of CCA and CCT is curried out step by step using the characteristics.
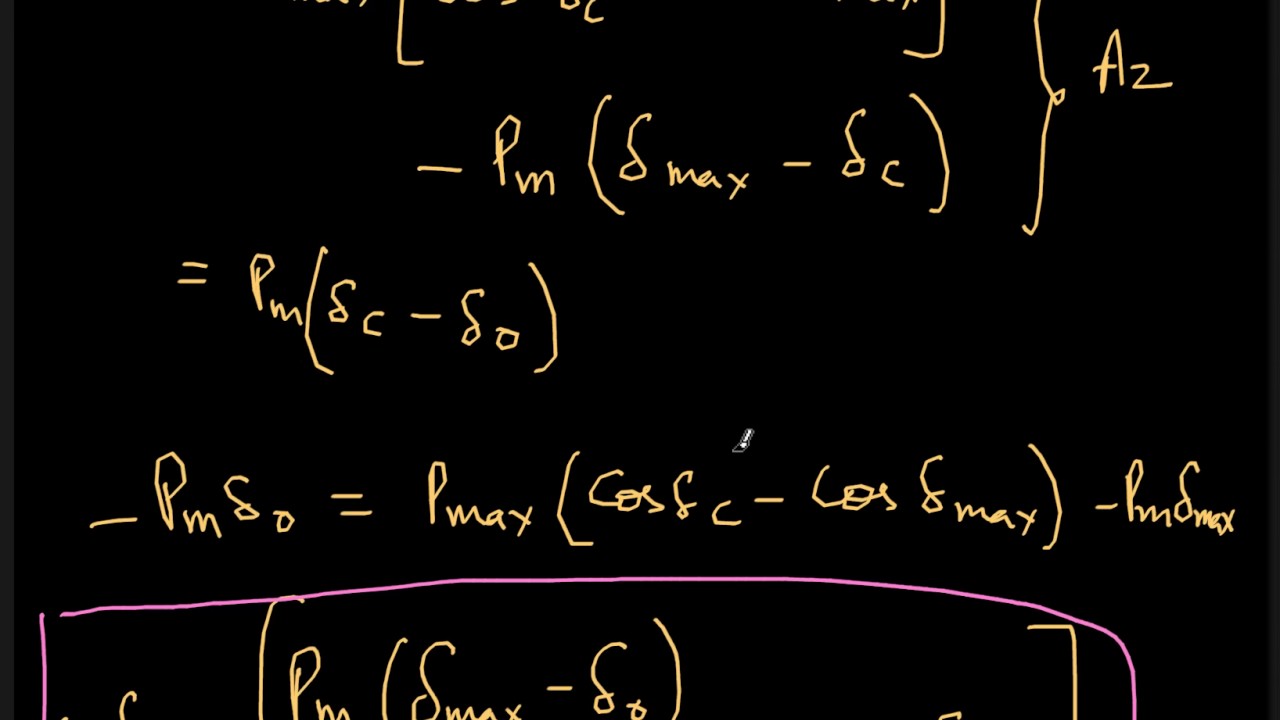
Critical Clearing Angle and Critical Clearing Time Numerical Example YouTube
Critical clearing angle. The greatest change in the load angle curve prior to clearing the fault without losing synchronism is known as the critical clearing angle. In other words, the system becomes unstable when a fault occurs because the load angle curve starts to rise. The CCA is the angle at which the fault is discovered and the system. First, equivalent Thevenin voltage and reactance before, during and after the fault are calculated respectively. Then, by applying the equal-area criteria, critical clearing angle can be obtained, and CFT can be calculated by improved Euler method. Finally, a four-bus network is used as a case study.
As the clearing angle δ c is increased, area A 1 increases and to find A 2 = A 1, δ 2 increases till it has a value δ max, the maximum allowable for stability. This case of critical clearing angle is shown in Fig. 12.31. Applying Equal Area Criterion in Power System to the case of critical clearing angle of Fig. 12.31, we can write. where. Power System StabilityCritical Clearing AngleCritical Clearing Time

Critical Clearing Angle with Loss of Transmission Capacity Numerical Example YouTube
The rotor angle at the critical clearing time is defined as the critical clearing angle δ G, cr, dc. 3.1.2 Analytical solution of critical clearing angle. A graphical interpretation of the power-angle relationships serves as a starting point in developing the analytical solution of the critical clearing angle. The critical clearing angle , is the maximum allowable change in the power angle δ before clearing the fault, without loss of synchronism. The time corresponding to this angle is called critical clearing time, .It can be defined as the maximum time delay that can be allowed to clear a fault without loss of synchronism.