Successive Differentiation is the process of differentiating a given function successively times and the results of such differentiation are called successive derivatives. The higher order differential coefficients are of utmost importance in scientific and engineering applications. SUCCESSIVE DIFFERENTIATION & LEIBNITZ'S THEOREM [The process of differentiating a function successively is called Successive Differentiation and the resulting derivatives are known as successive derivatives.] Commonly used notations for higher order derivatives of a function 1 Derivative: ′( ) or ′ or 2 Derivative: ′′( ) or 1 or ′′ or 2 or
Successive Differentiation OMG { Maths }
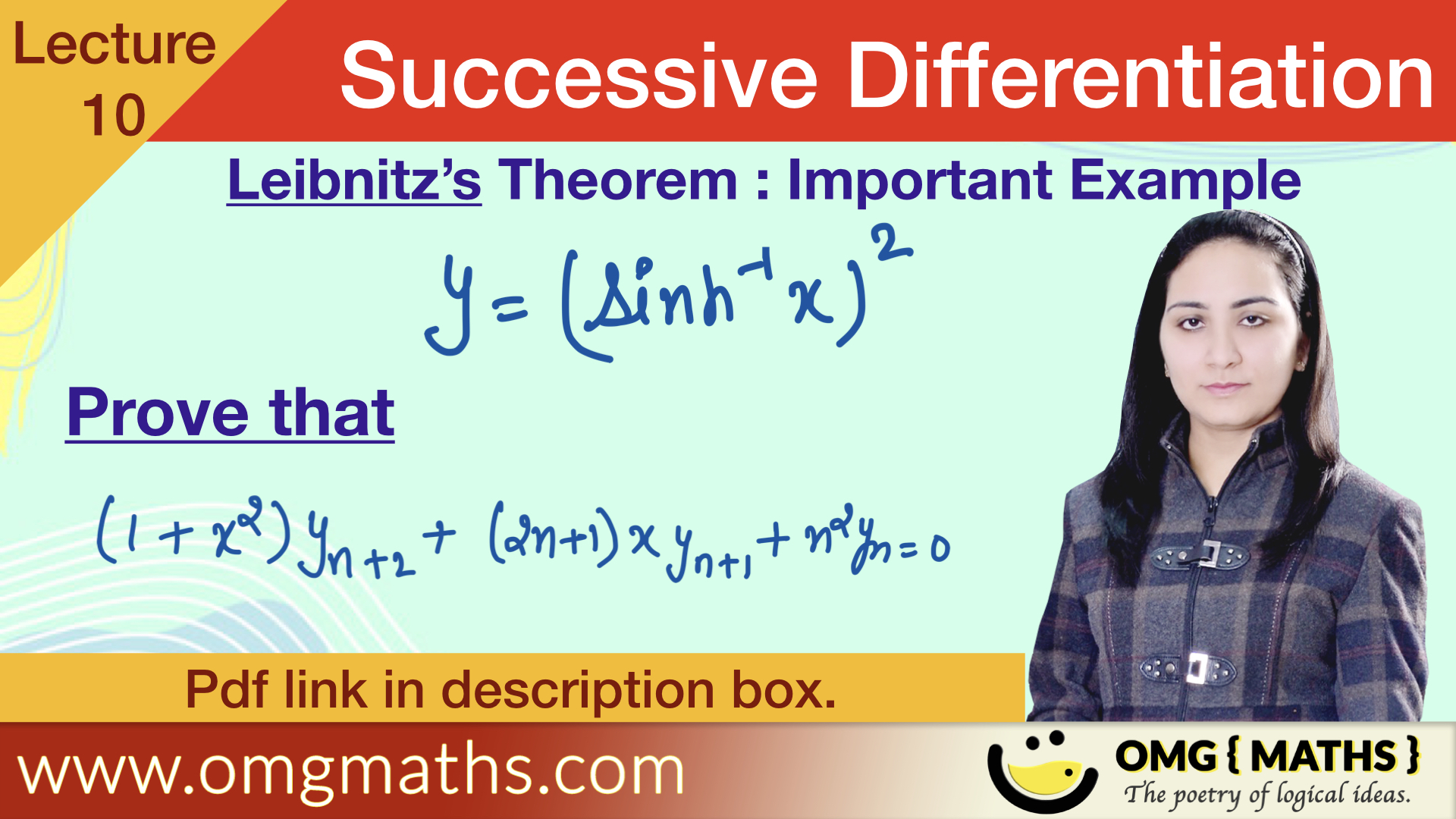
SUCCESSIVE DIFFERENTIATION Let f be a differentiable function on an interval I. Then the derivative f′ is a function of x and if f′ is differentiable at x, then the derivative of f′ at x is called second derivative of f at x. r. +. 1. From (4) we see that if the theorem is true for any value of n, it is also true for the next value of n. But. we have already seen that the theorem is true for n =1.Hence is must be true for n =2 and so for n =3, and so on. Thus the Leibnitz's theorem is true for all positive integral values of n. Example. Introduction Let f(x) be a differentiable function on an interval I. Then the derivative f0(x) is a function of x and if f0(x) is differentiable at x, then the derivative of f0(x) at x is called second derivative of f(x) at x and it is denoted by f00(x) or f(2)(x). Proceeding in this way the nth order derivative Successive Differentiation Definition: Let be an interval and We say is twice differentiable at if is differentiable on for some and the derivative function is differentiable at . In that case we define the second order derivative of at to be the derivative of the derivative function. It is also denoted by

Differentiation Rules PDF
2 Formulas for successive derivatives We have the following formulas for successive derivatives of composite functions with the exponential, or the logarithmic, inner function. Lemma 1. If f ∈ C∞(R) then the following formulas for the nth order (n = 1,2,3,.) derivatives hold dn dtn (f(e t)) = Xn k=1 ˆ n k ˙ f(k)(e )ekt, (6) dn dtn (f. [The process of differentiating a function successively is called Successive Differentiation and the resulting derivatives are known as successive derivatives.] Commonly used notations for higher order derivatives of a function Derivative: ′( ) or ′ or 2 Derivative: ′′( ) or or ′′ or 2 or = ( ) or 2 2 ⋮ Successive Differentiation Silvanus P. Thompson F.R.S. & Martin Gardner Chapter 332 Accesses Abstract Let us try the effect of repeating several times over the operation of differentiating a function. Begin with a concrete case. Chapter PDF Download to read the full chapter text Copyright information © 1998 Martin Gardner About this chapter Successive Differentiation. In: Calculus Made Easy: Being a Very-Simplest Introduction to those Beautiful Methods of Reckoning which are Generally called by the Terrifying names of the Differential Calculus and the Integral Calculus. Palgrave, London. https://doi.org/10.1007/978-1-349-00487-4_7 Download citation .RIS .ENW .BIB

1 Successive differentiation Matrices And Calculus Studocu
THE SUCCESSIVE DIFFERENTIATION METHOD FOR SOLVING BRATU EQUATION AND BRATU-TYPE EQUATIONS Published 2016 Mathematics In this work, we apply the successive differentiation method for solving the nonlinear Bratu problem and a variety of Bratu-type equations. Leibniz theorem of successive differentiation (see [1])is basically a generalization of the product rule of differentiation. In this article we will use the Leibniz's theorem of successive differen- tiation in order to construct and evaluate some important summations and a limit involving Euler-Mascheroni constant (see [4]) .
For that we mention the hypergeometric forms of some composite functions in section 2, with their proof in section 3, using the series rearrangement technique. Applications of these hypergeometric forms in successive differentiation (mentioned in section 4), are given in section 5. 2. derivatives of the exponential and logarithm functions came from the defini-tion of the exponential function as the solution of an initial value problem. To find the derivatives of the other functions we will need to start from the definition. An example: f(x) = x3 We begin by examining the calculation of the derivative of f(x) = x3 using

Successive Differentiation
Successive Differentiation Let us try the effect of repeating several times over the operation of differentiating a function (see here ). Begin with a concrete case. Let y = x5 y = x 5 . Successive differentiation is the process of differentiating a given function several times, and the results are called successive derivatives. In this article, let us learn more about successive differentiation. Derivative of a Function




