As mentioned at the beginning of this section, exponential functions are used in many real-life applications. The number e is often associated with compounded or accelerating growth, as we have seen in earlier sections about the derivative.Although the derivative represents a rate of change or a growth rate, the integral represents the total change or the total growth. The following is a list of integrals of exponential functions. For a complete list of integral functions, please see the list. Etisha Sharma, "Putting Forward Another Generalization Of The Class Of Exponential Integrals And Their Applications.," International Journal of Scientific Research in Mathematical and Statistical Sciences, Vol.10.
core pure 3 notes integrals involving exponentials
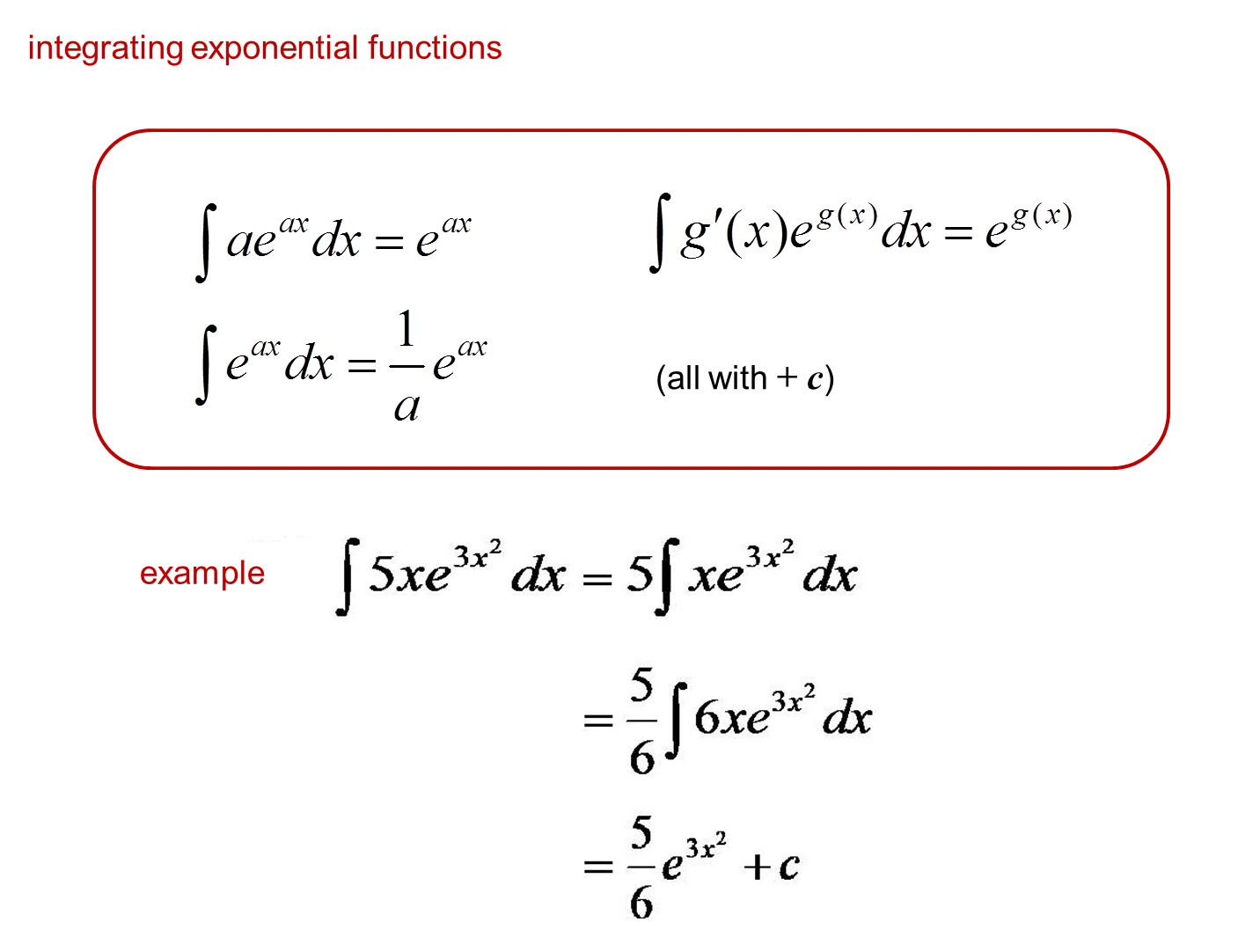
Rule: Integrals of Exponential Functions. Exponential functions can be integrated using the following formulas. ∫exdx = ex + C ∫axdx = ax lna + C. Example 5.6.1: Finding an Antiderivative of an Exponential Function. Find the antiderivative of the exponential function e − x. Solution. Exponential functions are those of the form \(f(x)=Ce^{x}\) for a constant \(C\), and the linear shifts, inverses, and quotients of such functions. Exponential functions occur frequently in physical sciences, so it can be very helpful to be able to integrate them. Nearly all of these integrals come down to two basic formulas: 5.6.2 Integrate functions involving logarithmic functions. Exponential and logarithmic functions are used to model population growth, cell growth, and financial growth, as well as depreciation, radioactive decay, and resource consumption, to name only a few applications. In this section, we explore integration involving exponential and. can be used, for example, the exponential integral EiHzL can be defined by the following formula (see the following sections for the corresponding series for the other integrals): EiHzL− 1 2 logHzL-log 1 z +â k=1 ¥zk kk! +ý. A quick look at the exponential integrals Here is a quick look at the graphics for the exponential integrals along.
Integration with exponential functions YouTube
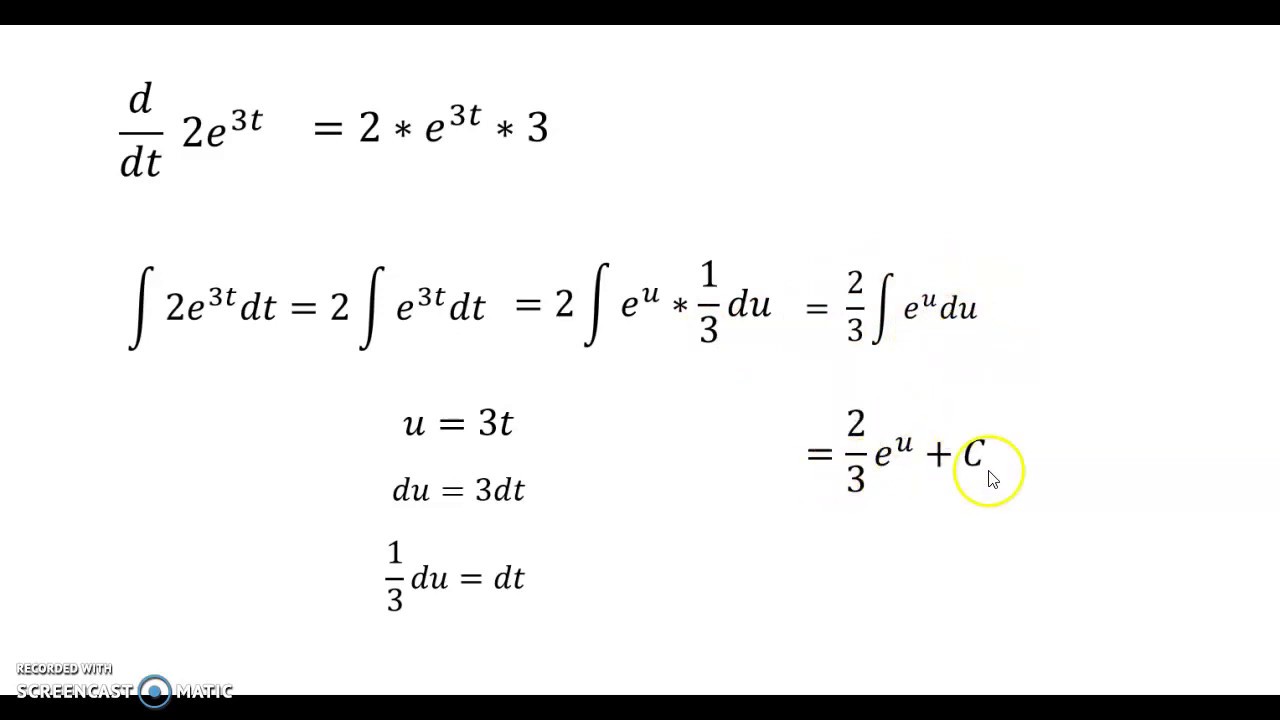
Let's rectify that here by defining the function f(x) = ax in terms of the exponential function ex. We then examine logarithms with bases other than e as inverse functions of exponential functions. Definition: Exponential Function. For any a > 0, and for any real number x, define y = ax as follows: y = ax = exlna. The following problems involve the integration of exponential functions. We will assume knowledge of the following well-known differentiation formulas : , and. is any positive constant not equal to 1 and is the natural (base ) logarithm of . These formulas lead immediately to the following indefinite integrals : Exponential Integral. where the retention of the notation is a historical artifact. Then is given by the integral. This function is implemented in the Wolfram Language as ExpIntegralEi [ x ]. The exponential integral is closely related to the incomplete gamma function by. Since the derivative of e^x is itself, the integral is simply e^x+c. The integral of other exponential functions can be found similarly by knowing the properties of the derivative of e^x.

Calculus Integration of Exponential Functions
The Derivative of the Exponential. We will use the derivative of the inverse theorem to find the derivative of the exponential. The derivative of the inverse theorem says that if f f and g g are inverses, then. g′(x) = 1 f′(g(x)). g ′ ( x) = 1 f ′ ( g ( x)). Let. f(x) = ln(x) f ( x) = ln ( x) then. f′(x) = 1 x f ′ ( x) = 1 x. Comments. The function $\mathop {\rm Ei}$ is usually called the exponential integral. Instead of by the series representation, for complex values of $ z $ ( $ x $ not positive real) the function $ \mathop {\rm Ei} ( z) $ can be defined by the integal (as for real $ x \neq 0 $); since the integrand is analytic, the integral is path-independent.
Let's look at an example in which integration of an exponential function solves a common business application. A price-demand function tells us the relationship between the quantity of a product demanded and the price of the product. In general, price decreases as quantity demanded increases. The marginal price-demand function is the. The exponential integrals , , , , , , and are defined for all complex values of the parameter and the variable . The function is an analytical functions of and over the whole complex ‐ and ‐planes excluding the branch cut on the ‐plane. For fixed , the exponential integral is an entire function of .

Calculus I Integrals of Exponential Functions YouTube
Well, to find the antiderivative (integral) of an exponential function, we will apply the same three steps, except instead of multiply, we will divide! Rewrite. Divide by the natural log of the base. Divide by the derivative of the exponent. ∫ a b x d x = a b x b ( ln a) + C. a: The base of the exponential function. the harder integral and the easier integral is a known term-that is the point. One note before starting: Integration by parts is not just a trick with no meaning. On the contrary, it expresses basic physical laws of equilibrium and force balance. It is a foundation for the theory of differential equations (and even delta functions).




