where e is the base of the natural logarithm, i is the imaginary unit, and cos and sin are the trigonometric functions cosine and sine respectively. This complex exponential function is sometimes denoted cis x ("cosine plus i sine"). The formula is still valid if x is a complex number, and is also called Euler's formula in this more general case. The basic relationship between the sine and cosine is given by the Pythagorean identity: where means and means This can be viewed as a version of the Pythagorean theorem, and follows from the equation for the unit circle.
Sin Cos Tan Triangle Calculator Cheapest Wholesalers, Save 52 jlcatj
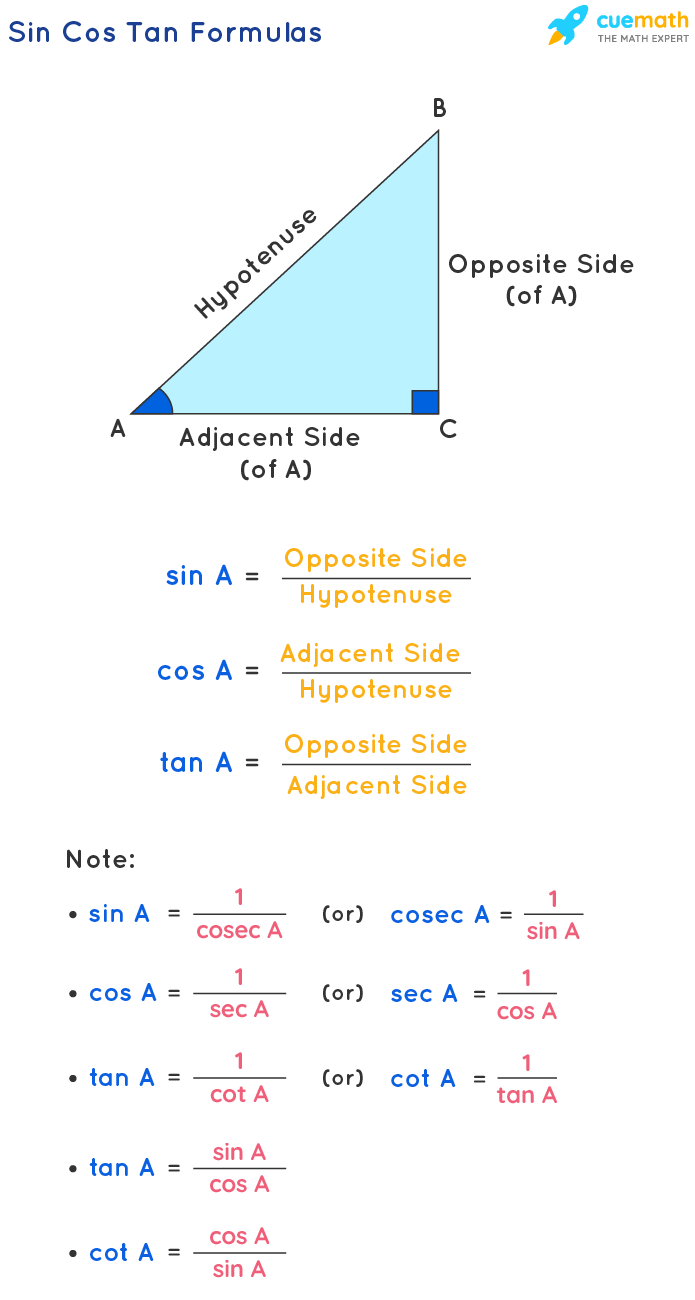
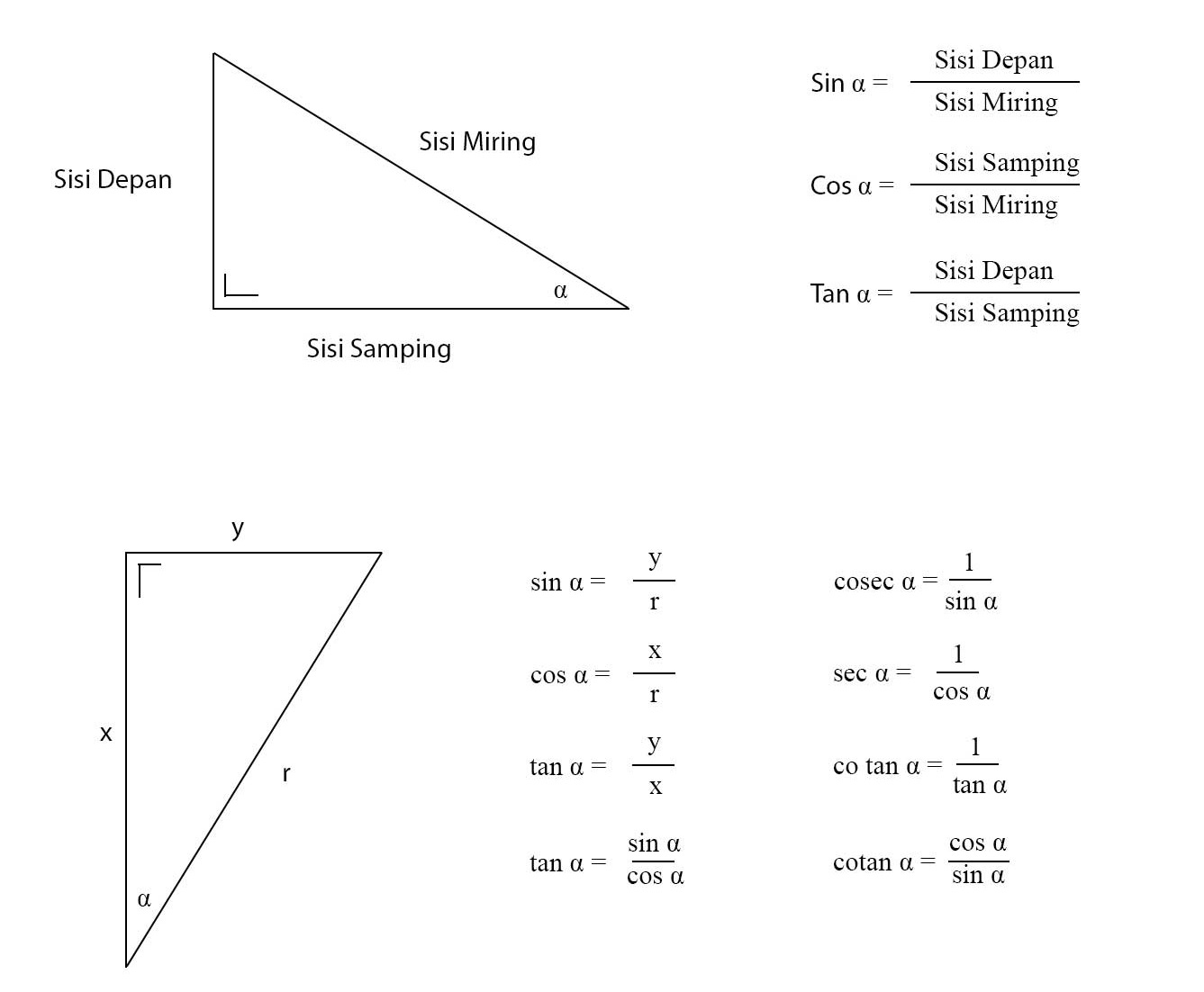
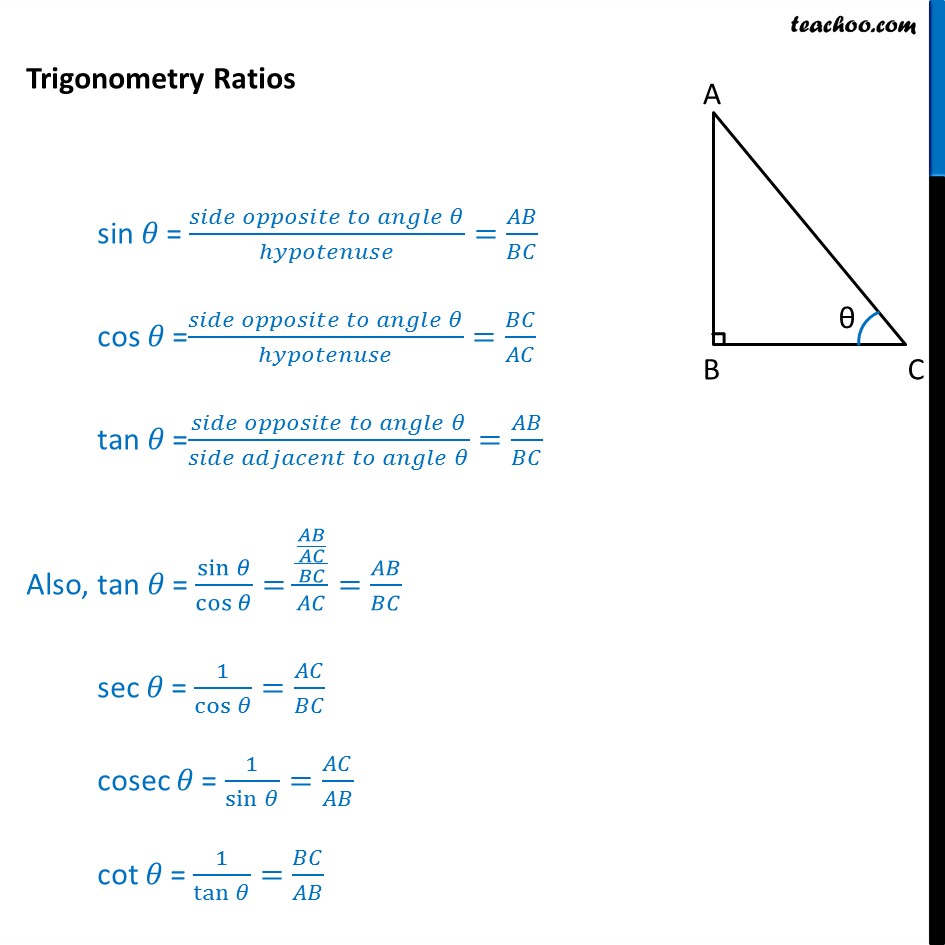
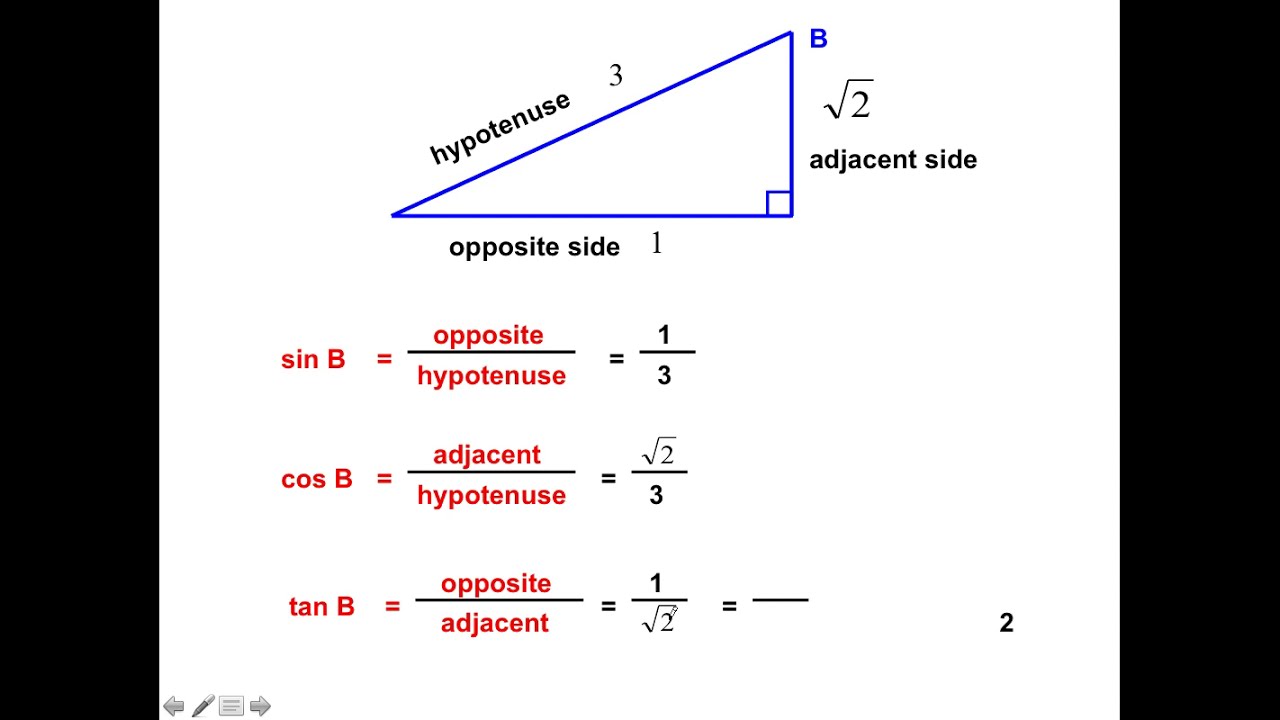
De nition (Cosine and sine). Given a point on the unit circle, at a counter-clockwise angle from the positive x-axis, cos is the x-coordinate of the point. sin is the y-coordinate of the point. The picture of the unit circle and these coordinates looks like this: Three trigonometric ratios Trigonometry involves three ratios - sine, cosine and tangent which are abbreviated to \ (\sin\), \ (\cos\) and \ (\tan\). The three ratios are calculated by. cis is a mathematical notation defined by cis x = cos x + i sin x, [nb 1] where cos is the cosine function, i is the imaginary unit and sin is the sine function. x is the argument of the complex number (angle between line to point and x-axis in polar form ). Sines Cosines Tangents Cotangents Pythagorean theorem Calculus Trigonometric substitution Integrals ( inverse functions) Derivatives v t e Basis of trigonometry: if two right triangles have equal acute angles, they are similar, so their corresponding side lengths are proportional.
Belajar Sin Cos Tan Rumus Dengan Mudah Panduan Lengkap Untuk Pemula
Trigonometry involves three ratios - sine, cosine and tangent which are abbreviated to \(\sin\), \(\cos\) and \(\tan\). The three ratios can be found by calculating the ratio of two sides of a. When we divide Sine by Cosine we get: sin (θ) cos (θ) = Opposite/Hypotenuse Adjacent/Hypotenuse = Opposite Adjacent = tan (θ) So we can say: tan (θ) = sin (θ) cos (θ) That is our first Trigonometric Identity. Cosecant, Secant and Cotangent We can also divide "the other way around" (such as Adjacent/Opposite instead of Opposite/Adjacent ): Purplemath What is an identity? In mathematics, an "identity" is an equation which is always true, regardless of the specific value of a given variable. An identity can be "trivially" true, such as the equation x = x or an identity can be usefully true, such as the Pythagorean Theorem's a2 + b2 = c2 MathHelp.com Need a custom math course? AboutTranscript. Euler's formula is eⁱˣ=cos (x)+i⋅sin (x), and Euler's Identity is e^ (iπ)+1=0. See how these are obtained from the Maclaurin series of cos (x), sin (x), and eˣ. This is one of the most amazing things in all of mathematics! Created by Sal Khan.
What are sin cos tan? Chapter 8 Class 10 Trignometric Ratios
cos x - cos y = -2 sin( (x - y)/2 ) sin( (x + y)/2 ) Trig Table of Common Angles; angle 0 30 45 60 90; sin ^2 (a) 0/4 : 1/4 : 2/4 : 3/4 : 4/4 : cos ^2 (a) 4/4 : 3/4 : 2/4 : 1/4 : 0/4 : tan ^2 (a) 0/4 : 1/3 : 2/2 : 3/1 : 4/0 ; Given Triangle abc, with angles A,B,C; a is opposite to A, b opposite B, c opposite C: Free math problem solver answers your trigonometry homework questions with step-by-step explanations.
Want to learn more about the law of cosines? Check out this video. Practice set 1: Solving triangles using the law of sines This law is useful for finding a missing angle when given an angle and two sides, or for finding a missing side when given two angles and one side. Example 1: Finding a missing side Let's find A C in the following triangle: The three basic trigonometric functions are: Sine (sin), Cosine (cos), and Tangent (tan). What is trigonometry used for? Trigonometry is used in a variety of fields and applications, including geometry, calculus, engineering, and physics, to solve problems involving angles, distances, and ratios. Show more Why users love our Trigonometry Calculator
Rumus Sin, Cos, Tan Dan Tabel
Sines Cosines Tangents Cotangents Pythagorean theorem Calculus Trigonometric substitution Integrals ( inverse functions) Derivatives v t e In mathematics, sine and cosine are trigonometric functions of an angle. GCSE Trigonometry - Intermediate & Higher tier - WJEC Sin, cos and tan Trigonometric relationships are very important in the construction and planning industry and allow precise calculation of.




