Brownian motion is the random motion of particles suspended in a medium (a liquid or a gas ). [2] This motion pattern typically consists of random fluctuations in a particle's position inside a fluid sub-domain, followed by a relocation to another sub-domain. Each relocation is followed by more fluctuations within the new closed volume. The term "classical Brownian motion" describes the random movement of microscopic particles suspended in a liquid or gas.

IGCSE Physics Unit 2 Thermal physics
The goal of this experiment is to show real Brownian motion using a microscope. Theory In 1827, a Scottish botanist Robert Brown (1773-1857, Fig. 1) observed a chaotic motion of tiny particles ejected from pollen seeds suspended in water for the first time. Produced by the National STEM Learning Centre and Network and the Institute of Physics, this video illustrates how to show the movement of particles by Brown. Definition (#1.). A Brownian motion or Wiener process (Wt)t 0 is a real-valued stochastic process such that W0 = 0; Independent increments: the random variables Wv Wu, Wt Ws are independent whenever u v t (so the intervals (u;v), (s;t) are disjoint.) Brownian motion refers to the indefinitely continuing complex motion of particles, or granules, in a dilute emulsion or colloidal solution.Perrin's experiments were designed to yield theory-mediated measurements of aspects of that motion from features of it that could be observed in a sufficiently high-powered microscope.

1.3 Diffusion & Brownian Motion Qatar Science
Produced by the Institute of Physics and the National STEM Learning Centre and Network (https://www.stem.org.uk/), this video illustrates how to show the mov. The operations of averaging and taking the time derivative commute, so we can write the equation: m 2 d2 dt2 x2¯ ¯¯¯¯ + 3παη d dtx2¯ ¯¯¯¯ = KBT. (1.12.9) (1.12.9) m 2 d 2 d t 2 x 2 ¯ + 3 π α η d d t x 2 ¯ = K B T. To solve this differential equation, write d dtx2¯ ¯¯¯¯ = y. d d t x 2 ¯ = y. The equation becomes: This paper will show how video recordings of the Brownian motion of tiny particles may be made, describe a classroom demonstration, cite a reported experiment designed to show the random nature of Brownian motion, and suggest a student experiment to discover the distance that a tiny particle travels as a function of time. KEYWORDS (Audience): Brownian motion is the perpetual irregular motion exhibited by small particles immersed in a fluid. Such random motion of the particles is produced by statistical fluctuations in the collisions they suffer with the molecules of the surrounding fluid. Brownian motion of particles in a fluid (like milk particles in water) can be observed under a microscope. Here we describe a simple experimental.
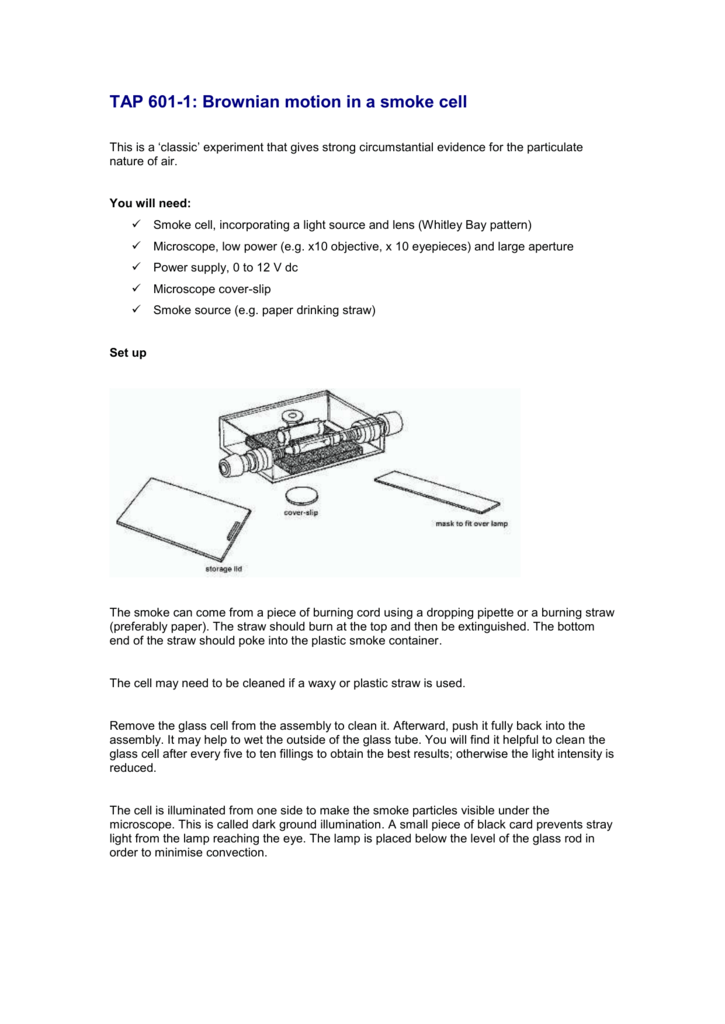
TAP 6011 Brownian motion in a smoke cell
Well, one experiment which adds evidence to support this 'kinetic' theory is called 'Brownian Motion'. To set up this experiment, we need: a glass 'cell'. smoke from a glowing splint. a microscope with a light to illuminate the smoke particles. The smoke is trapped in the glass cell and the microscope is used to observe the motion of the smoke. An alternative: use a visualiser with a data projector and screen to enable students to observe Brownian motion in a suspension containing tiny polystyrene spheres. This experiment was safety-tested in March 2005. This video shows how Brownian motion can be observed in a suspension containing micrometre diameter polystyrene spheres.
Brownian motion refers to the random motions of small particles under thermal excitation in solution first described by Robert Brown (1827), 1 who with his microscope observed the random, jittery spatial motion of pollen grains in water. This phenomenon is intrinsically linked with diffusion. Diffusion is the macroscopic realization of the. Brownian motion is considered a Gaussian process and a Markov process with continuous path occurring over continuous time. What Is Brownian Motion? Because the movements of atoms and molecules in a liquid and gas is random, over time, larger particles will disperse evenly throughout the medium.

PPT Brownian Motion PowerPoint Presentation, free download ID3443530
Description of Albert Einstein's theory of Brownian motion and how he derived the size of atoms. © MinutePhysics ( A Britannica Publishing Partner ) Learn about Albert Einstein's theory of Brownian motion and how he derived the size of atoms based on how much the Brownian particles move Procedure: Place the microslide with the desired density of microspheres under the microscope. Using the 100 power lens you may observe the Brownian motion. Poisson Distribution. THIS WILL TAKE 2 TO 3 DAYS ADVANCED NOTICE: Prepare a microslide with a high density solution of microspheres.




