x = a cos ty = b sin t. t is the parameter, which ranges from 0 to 2π radians. This equation is very similar to the one used to define a circle, and much of the discussion is omitted here to avoid duplication. See Parametric equation of a circle as an introduction to this topic. The only difference between the circle and the ellipse is that in. 7. The parametric equation of an ellipse is. x = a cos t y = b sin t. It can be viewed as x coordinate from circle with radius a, y coordinate from circle with radius b. How to prove that it's an ellipse by definition of ellipse (a curve on a plane that surrounds two focal points such that the sum of the distances to the two focal points is.
Parametric equation Q No 1 Equation of Ellipse YouTube
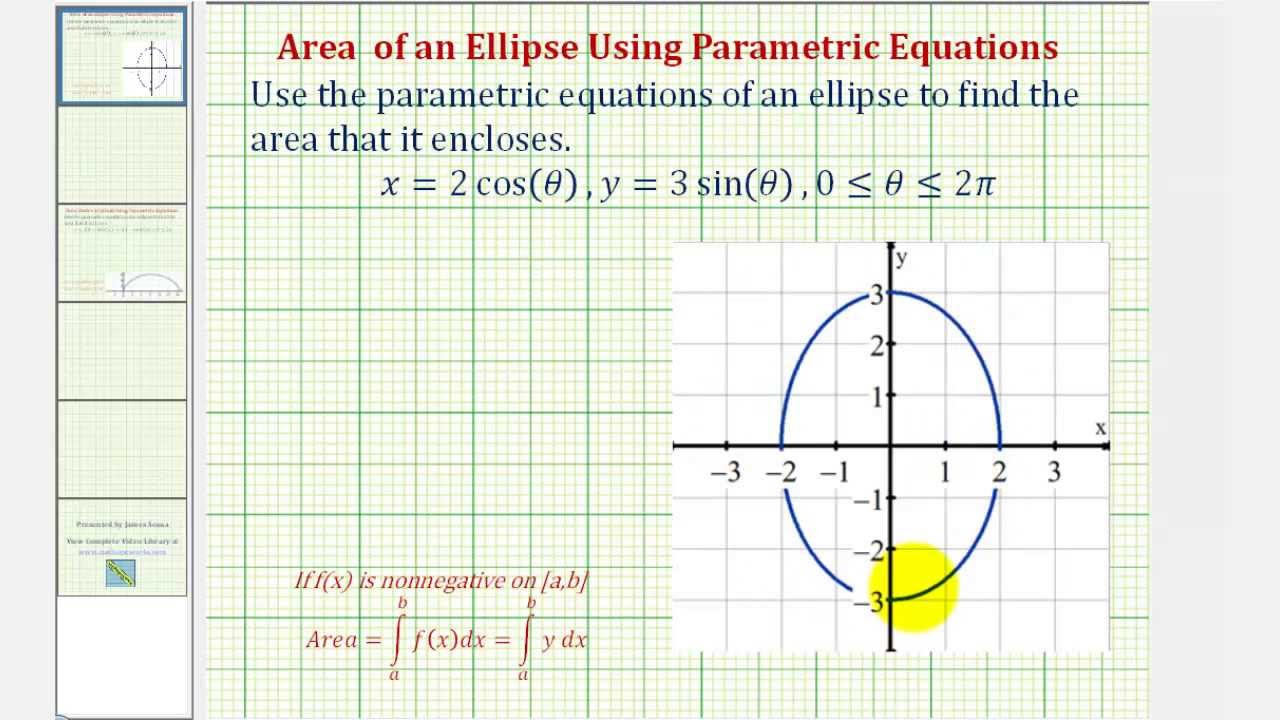
This video is a part of the Ellipse playlist: https://www.youtube.com/playlist?list=PLLLfkE_CWWawCB50B0g3ooPIIY72kDAQSSee more about ellipse: https://math-st. The parametric equations limit \(x\) to values in \((0,1]\), thus to produce the same graph we should limit the domain of \(y=1-x\) to the same.. This final equation should look familiar -- it is the equation of an ellipse! Figure 9.26 plots the parametric equations, demonstrating that the graph is indeed of an ellipse with a horizontal. When given an equation for an ellipse centered at the origin in standard form, we can identify its vertices, co-vertices, foci, and the lengths and positions of the major and minor axes in order to graph the ellipse. See Example \(\PageIndex{3}\) and Example \(\PageIndex{4}\). Given the ellipse. x2 a2 + y2 b2 = 1 x 2 a 2 + y 2 b 2 = 1. a set of parametric equations for it would be, x =acost y =bsint x = a cos t y = b sin t. This set of parametric equations will trace out the ellipse starting at the point (a,0) ( a, 0) and will trace in a counter-clockwise direction and will trace out exactly once in the range 0 ≤ t.
Integration Application Area Using Parametric Equations Ellipse YouTube
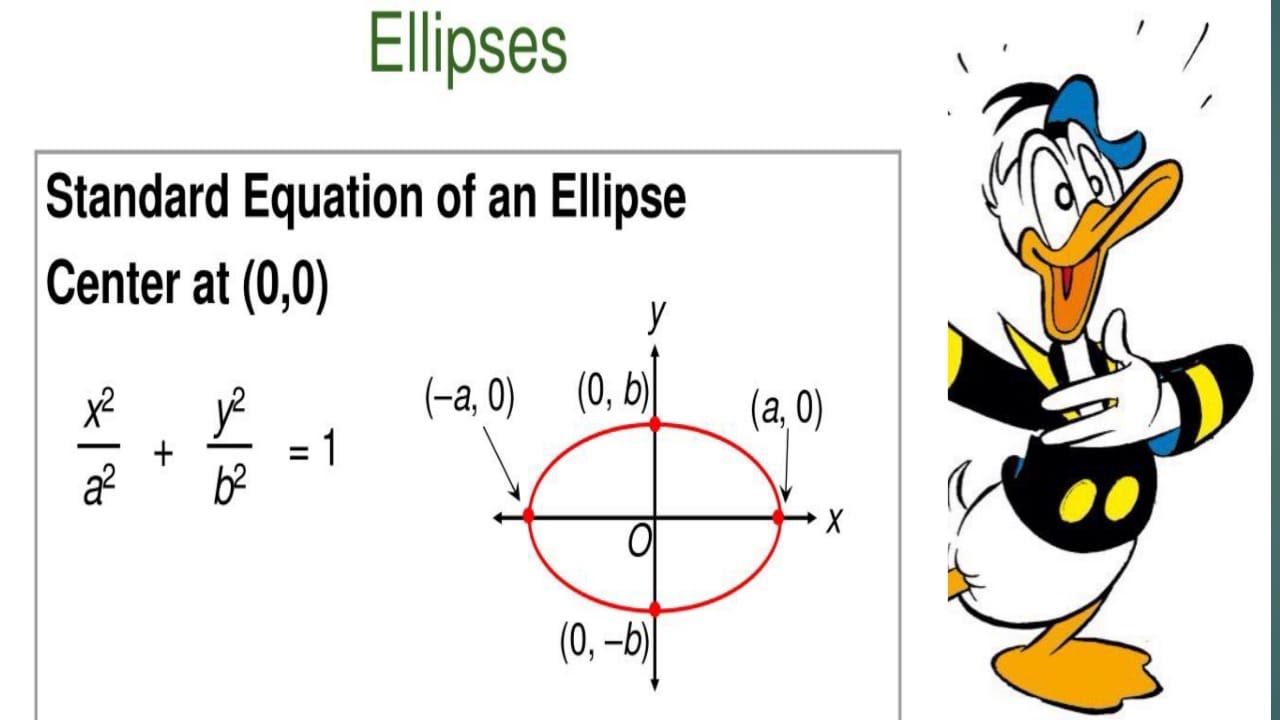
Parametric form. In parametric form, the equation of an ellipse with center (h, k), major axis of length 2a, and minor axis of length 2b, where a > b and θ is an angle in standard position can be written using one of the following sets of parametric equations. when the major axis is horizontal. x = h + a·cos(θ), y = k + b·sin(θ) The parametric equation of an ellipse centered at \((0,0)\) is \[f(t) = a\cos t, \quad g(t) = b\sin t.\] Our approach is to only consider the upper half, then multiply it by two to get the area of the entire ellipse. First, we need to find the left and right bounds in terms of \(t\), such that Since the parametric equation is only defined for \(t > 0\), this Cartesian equation is equivalent to the parametric equation on the corresponding domain.. This is a Cartesian equation for the ellipse we graphed earlier. Parameterizing Curves. While converting from parametric form to Cartesian can be useful, it is often more useful to. An ellipse is the locus of a point whose sum of the distances from two fixed points is a constant value. The two fixed points are called the foci of the ellipse, and the equation of the ellipse is x2 a2 + y2 b2 = 1 x 2 a 2 + y 2 b 2 = 1. Here. a is called the semi-major axis.

How to Write the Parametric Equations of an Ellipse in Rectangular Form YouTube
Parametric equation of ellipse. By Martin McBride, 2020-09-14 Tags: ellipse major axis minor axis Categories: coordinate systems pure mathematics. The parametric equation of an ellipse is: $$ \begin{align} x = a \cos{t}\newline y = b \sin{t} \end{align} $$ Understanding the equations. This is the equation of a horizontal ellipse centered at the origin, with semimajor axis 4 and semiminor axis 3 as shown in the following graph.. Earlier in this section, we looked at the parametric equations for a cycloid, which is the path a point on the edge of a wheel traces as the wheel rolls along a straight path. In this project we.
1.3.1 Ellipse Parametric Equation. x ( t) = r cos ( θ) + h y ( t) = r sin ( θ) + k. The conic section most closely related to the circle is the ellipse. We have been reminded in class that the general equation of an ellipse is given by. x 2 a 2 + y 2 b 2 = 1. An ellipse is a curve that is the locus of all points in the plane the sum of whose distances r_1 and r_2 from two fixed points F_1 and F_2 (the foci) separated by a distance of 2c is a given positive constant 2a (Hilbert and Cohn-Vossen 1999, p. 2). This results in the two-center bipolar coordinate equation r_1+r_2=2a, (1) where a is the semimajor axis and the origin of the coordinate system.
Parametric Equations of Ellipse Example 1 椭圆参数方程 YouTube
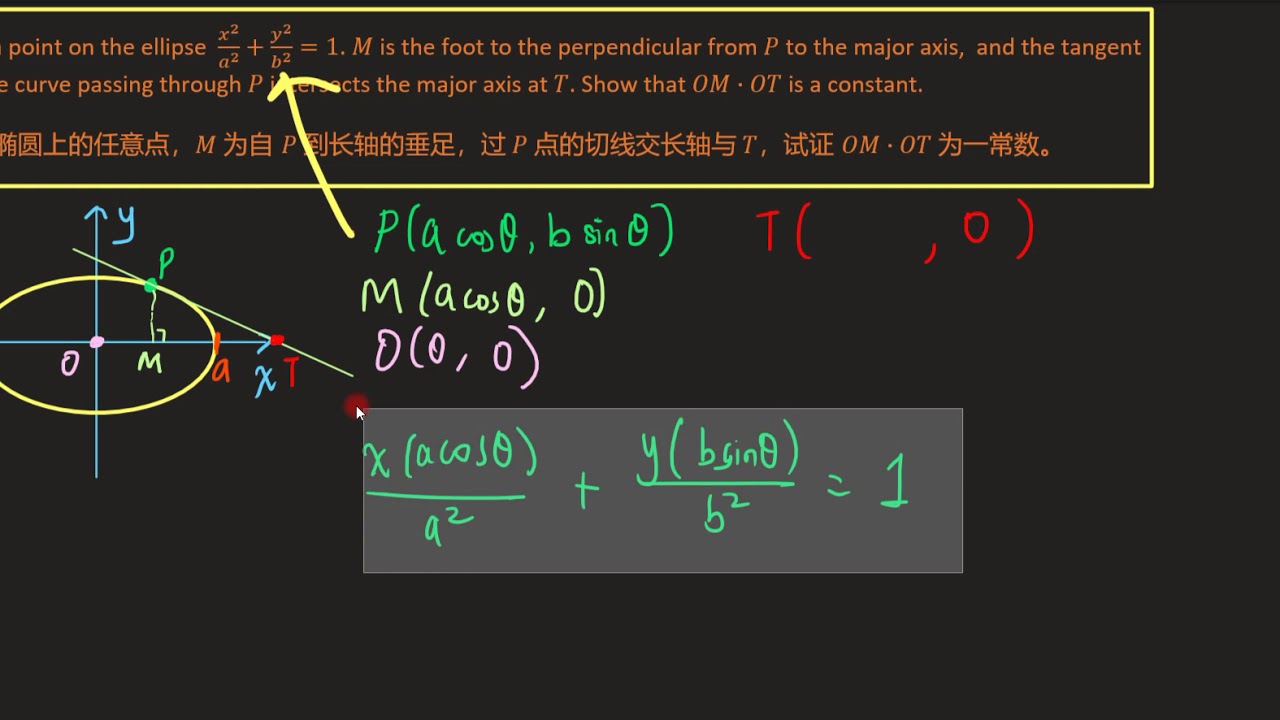
Now from P draw PM perpendicular to the major axis of the ellipse and produced MP cuts the auxiliary circle x2 2 + y2 2 = a2 2 at Q. Join the point C and Q. Again, let ∠XCQ = ф. The angle ∠XCQ = ф is called the eccentric angle of the point P on the ellipse. The major axis of the ellipse x2 a2 x 2 a 2 + y2 b2 y 2 b 2 = 1 is AA' and its. Solution: The equation of the ellipse is: The general equation of ellipse is: On comparison: Hence: The length of the major axis = 2a =8. The length of the minor axis = 2b = 6. Example 2: The length of the semi-major and semi-minor axis of an ellipse is 4 cm and 2 cm respectively.




