We should learn it like sin 0° = 0 sin 30° = 1/2 sin 45° = 1/√2 sin 60° = √3/2 sin 90° = 1 So, our pattern will be like 0, 1/2, 1/√2, √3/2, 1 Tired of ads? Get Ad-free version of Teachoo for ₹ 999 ₹499 per month For cos For memorising cos 0°, cos 30°, cos 45°, cos 60° and cos 90° Cos is the opposite of sin. We should learn it like When writing about 30 60 90 triangle, we mean the angles of the triangle, that are equal to 30°, 60° and 90°. Assume that the shorter leg of a 30 60 90 triangle is equal to a. Then: The second leg is equal to a√3; The hypotenuse is 2a; The area is equal to a²√3/2; and The perimeter equals a (3 + √3).
Trigonometry Table Up To 360
Answer . According to the property of cofunctions, sin 30° is equal to cos 60°. sin 30° = ½. On the other hand, you can see that directly in the figure above. Problem 1. Evaluate sin 60° and tan 60°. To see the answer, pass your mouse over the colored area. To cover the answer again, click "Refresh" ("Reload"). Google Classroom Learn to find the sine, cosine, and tangent of 45-45-90 triangles and also 30-60-90 triangles. Until now, we have used the calculator to evaluate the sine, cosine, and tangent of an angle. However, it is possible to evaluate the trig functions for certain angles without using a calculator. Step 1: Create a table with the top row listing the angles such as 0°, 30°, 45°, 60°, 90°, and write all trigonometric functions in the first column such as sin, cos, tan, cosec, sec, cot. Step 2: Determine the value of sin To determine the values of sin, divide 0, 1, 2, 3, 4 by 4 under the root, respectively. See the example below. Trigonometry. Trigonometry is a branch of mathematics concerned with relationships between angles and ratios of lengths. The field emerged in the Hellenistic world during the 3rd century BC from applications of geometry to astronomical studies. The Greeks focused on the calculation of chords, while mathematicians in India created the earliest.

give the chart of trigonometry table and values of sin 30 60 90 etc Brainly.in
This trigonometry video tutorial provides a basic introduction into 30-60-90 triangles. It explains how to evaluate trigonometric functions such as sine and. Free math problem solver answers your trigonometry homework questions with step-by-step explanations. The value of Sin(30) holds significance in the analysis of special triangles like the 30-60-90 triangle and in geometric calculations. This is where Sin(30) comes into play. The value of the sine of the 30-degree angle, mathematically expressed as Sin(30), is equal to 1/2. This means that the short side will be half the length of the hypotenuse. Exact trigonometric ratios for 0°, 30°, 45°, 60° and 90° The trigonometric ratios for the angles 30°, 45° and 60° can be calculated using two special triangles. An equilateral.

sin60 表 Chqboks
2:40 Sal says the ratio is 1: square root of 3 : 2. Can someone explain this please? • ( 21 votes) Christopher 11 years ago They're the same thing, it's just that the former is expressed in terms of x (which is the hypotenuse). The basic 30-60-90 triangle ratio is: Side opposite the 30° angle: $x$ Side opposite the 60° angle: $x * √3$ Side opposite the 90° angle: $2x$ For example, a 30-60-90 degree triangle could have side lengths of: 2, 2√3, 4 7, 7√3, 14 √3, 3, 2√3 (Why is the longer leg 3?
How to use the trig ratios of special angles to find exact values of expressions involving sine, cosine and tangent values of 0, 30, 45, 60 and 90 degrees? Example: Determine the exact values of each of the following: a) sin30°tan45° + tan30°sin60°. b) cos30°sin45° + sin30°tan30°. Show Video Lesson. . I mean to say that sin 0 = cos 90

sin 30+ sin 60+sin 90÷ cos 30 + cos 60 + cos 90 Brainly.in
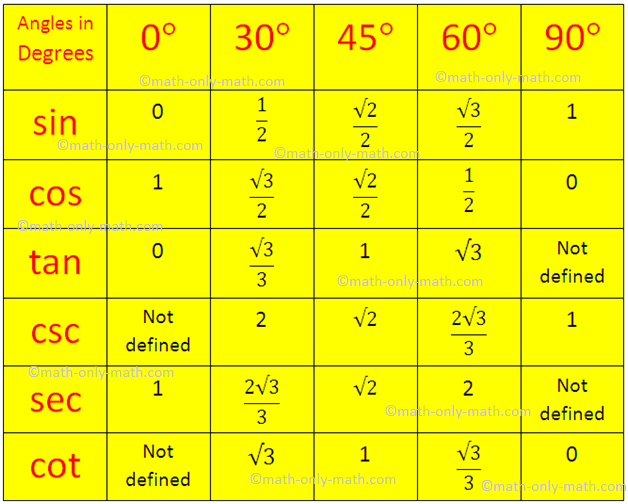
Trigonometric Table. A trigonometric table is a table that lists the values of the trigonometric functions for various standard angles such as 0°, 30°, 45°, 60°, and 90°. Trigonometric table comprises trigonometric ratios - sine, cosine, tangent, cosecant, secant, cotangent. These ratios, in short, are written as sin, cos, tan, cosec, sec, and cot. The sine of 30 degrees (sin 30°) can be calculated using the trigonometric values of special angles. In this case, 30 degrees is a special angle because it is one of the angles in a 30-60-90 degree right triangle. In a 30-60-90 triangle, the sides are in the ratio 1:√3:2.




