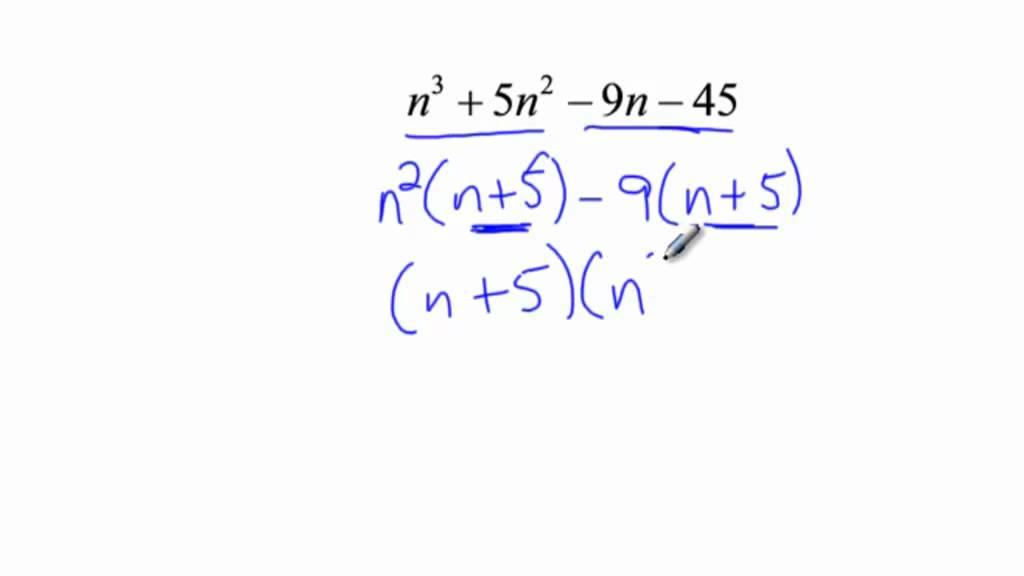Whenever you factor a polynomial (cubic or otherwise), you are finding simpler polynomials whose product equals the original polynomial. Each of these simpler polynomials is considered a factor of the original polynomial. For example, the binomial x ² - 100 has two factors (x + 10) and (x-10). Why? 1 Group the polynomial into two sections. Grouping the polynomial into two sections will let you attack each section individually. [1] Say we're working with the polynomial x 3 + 3x 2 - 6x - 18 = 0. Let's group it into (x 3 + 3x 2) and (- 6x - 18) 2 Find what's the common in each section. Looking at (x 3 + 3x 2 ), we can see that x 2 is common.

Factoring By Grouping Factoring By Grouping X^3
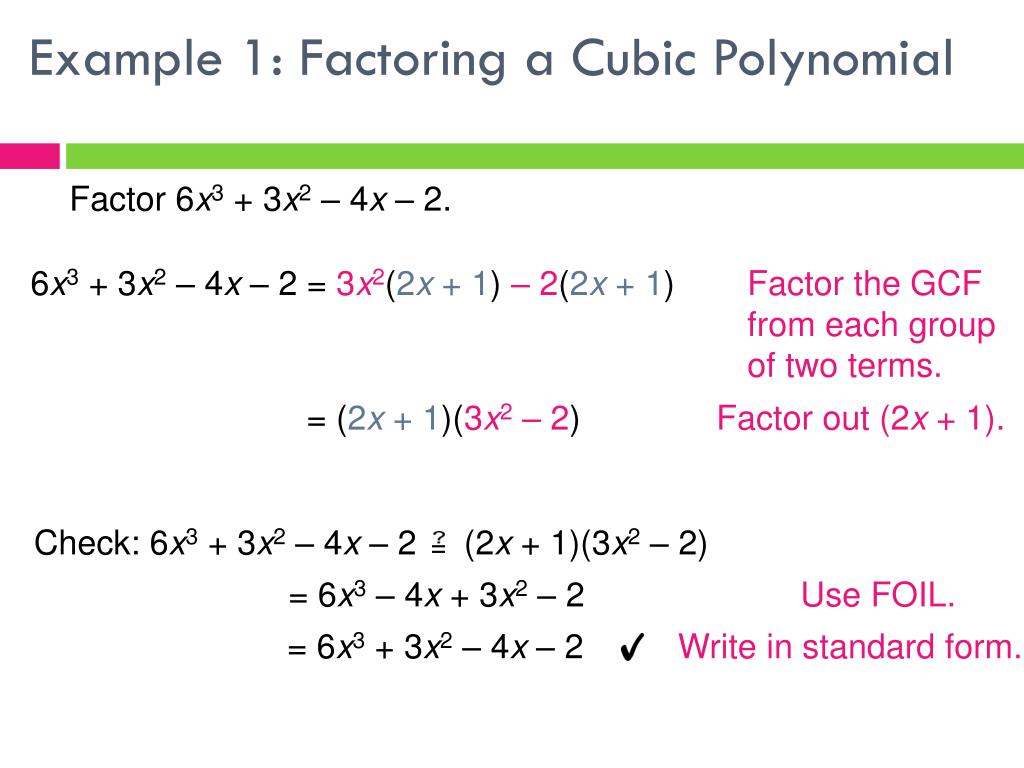
Factoring cubic polynomials is a process of finding the factors of the cubic polynomials. We can find the factors of a cubic polynomial using different methods such as long division, trial and error method, etc. The factors can be linear, quadratic, or cubic (if it does not have any roots). Factoring Cubic Polynomials Brody Acquilano , Patrick Corn , Ahmed Kamel , and 1 other contributed A cubic polynomial is a polynomial of the form f (x)=ax^3+bx^2+cx+d, f (x) = ax3 +bx2 +cx+ d, where a\ne 0. a = 0. If the coefficients are real numbers, the polynomial must factor as the product of a linear polynomial and a quadratic polynomial. Factoring by Grouping This is by far the nicest method of the two, but it only works in some cases. Consider the polynomial p(x) = x3 4x2 + 3x 12: We group the rst two terms and the last two terms together: p(x) = (x3 4x2) + (3x 12) and then we pull out the common factors: p(x) = x2(x 4) + 3(x 4): Notice now that these two terms now have x The goal of this free guide on how to factor polynomials is to give you plenty of step-by-step practice with factoring polynomials—including polynomials with 4 terms (cubic polynomials)—so that can become more comfortable with factoring all kinds of polynomials.
PPT 6.5 Factoring Cubic Polynomials PowerPoint Presentation, free download ID2795627
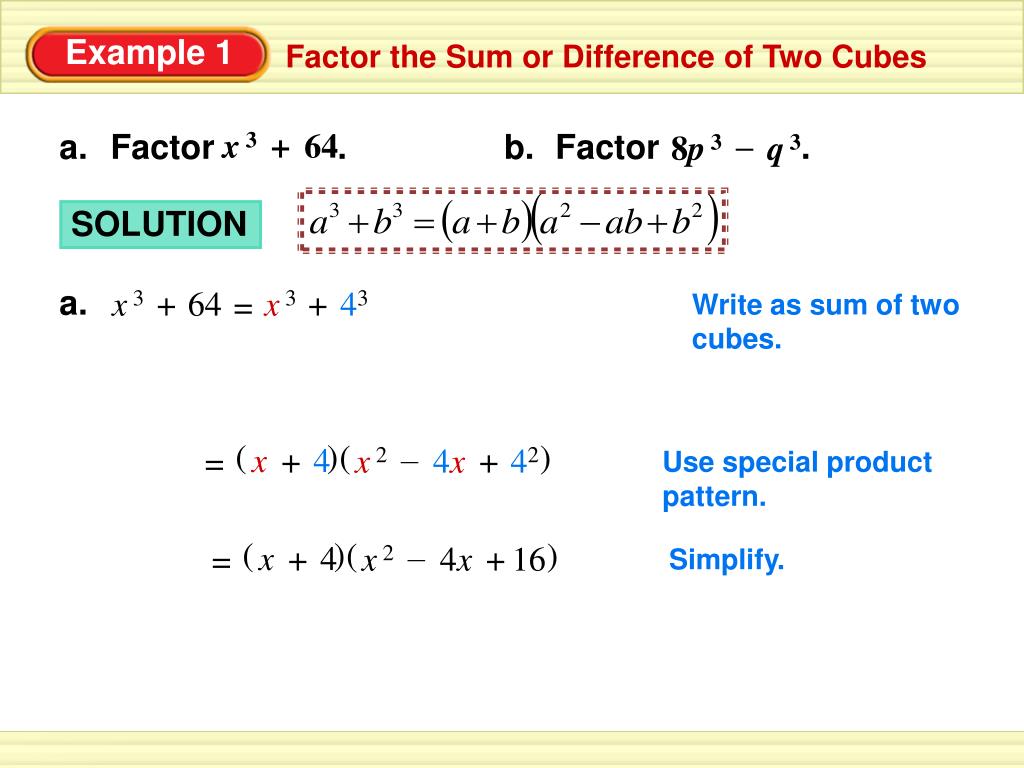
This algebra 2 and precalculus video tutorial explains how to factor cubic polynomials by factoring by grouping method or by listing the possible rational zeros of the polynomial and then. Factoring difference of cubes (video) | Khan Academy Algebra (all content) Course: Algebra (all content) > Unit 10 Factoring difference of cubes Math > Algebra (all content) > Polynomial expressions, equations, & functions > Advanced polynomial factorization methods © 2024 Khan Academy Terms of use Privacy Policy Cookie Notice The three methods we use for factoring a cubic polynomial are splitting terms using the ad-method, finding a factor by applying the rational root theorem, and cubic formulas for sum, difference, etc. Jump to Questions Irreducible Polynomials Polynomials like 2x + 1 or 3x 2 − x + 1 cannot be factorized. These are irreducible polynomials. Factoring a Cubic Polynomial - Algebra I BroandSisMathClub 18.4K subscribers Subscribe Subscribed 4.8K views 8 years ago In this video, you will learn how to factor a cubic polynomial. A.
How To Factor A Cubic Polynomial Cubic Equation Wikipedia / In this video we learn a more
About Transcript Factoring higher degree polynomials involves breaking down complex expressions into simpler parts. This process includes identifying common factors, using the distributive property, and recognizing perfect squares. The first step to factoring a cubic polynomial in calculus is to use the factor theorem. The factor theorem holds that if a polynomial p (x) is divided by ax - b and you have a remainder of 0 when it's expressed as p (b/a), then ax - b is a factor. It's a roundabout way of saying that if an expression divides evenly into a polynomial.
If you are factoring a polynomial and run into an irreducible quadratic, just leave it alone. The irreducible quadratic would be considered one of the factors of the polynomial. Factoring Cubic Functions.. Example \(\PageIndex{12}\): Factoring a Cubic Function. Completely factor the function \(f(x)=x^3+8x^2+21x+18\). 7 Answers Sorted by: 11 By the Rational Zero Theorem all the rational roots of x3 − 12x + 9 x 3 − 12 x + 9 must have a numerator which is a factor of 9 9 and a denominator which is a factor of 1 1. Therefore they have to be of the form 9 1 = 9 9 1 = 9 or 3 1 = 3 3 1 = 3. Let f(x) =x3 − 12x + 9 f ( x) = x 3 − 12 x + 9.
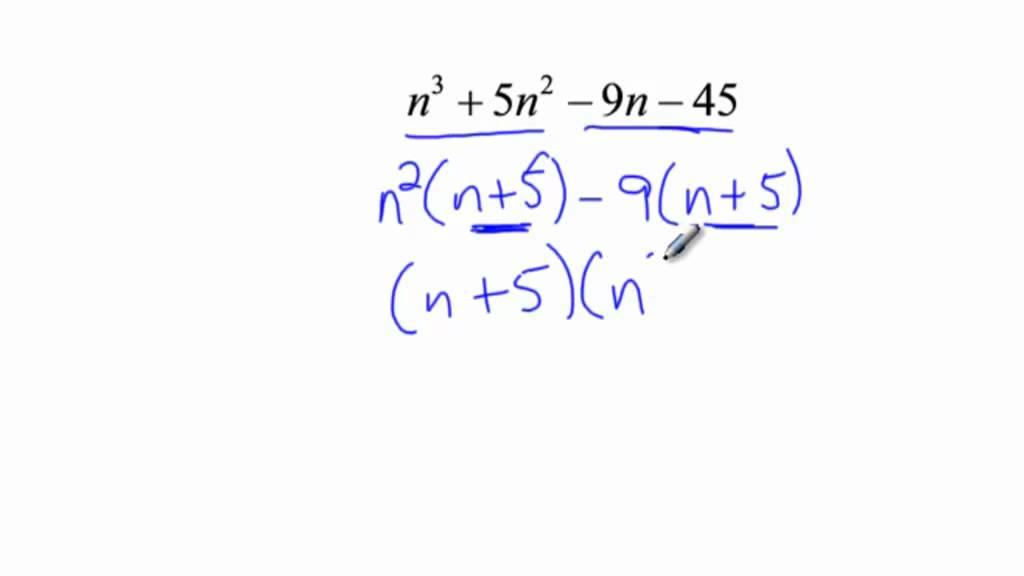
How To Factor Cubic Polynomials How to Solve Equations that are Not Perfectly Cubed Video
A tutorial on how to factorise polynomials of degree 3 (cubic functions). This video will cover the more popular of the two methods: the "kx" method. Every cubic polynomial will have 3 factors. To find those factors, we follow the following steps. Step 1 : We can find one linear factor of the given cubic polynomial using synthetic division. Step 2 : At the end of the first step, we will have quadratic factors. By factoring the quadratic equation, we can get other two factors.