Video transcript. Welcome to the presentation on quadratic inequalities. Before we get to quadratic inequalities, let's just start graphing some functions and interpret them and then we'll slowly move to the inequalities. Let's say I had f of x is equal to x squared plus x minus 6. Graphing Quadratic Inequalities. (or substitute <, ≥ <, ≥ or ≤ ≤ for > > ) represents a region of the plane bounded by a parabola . To graph a quadratic inequality, start by graphing the parabola. Then fill in the region either above or below it, depending on the inequality. If the inequality symbol is ≤ ≤ or ≥ ≥ , then the.

4.6 Graphing Quadratic Inequalities Ms. Zeilstra's Math Classes
Explore math with our beautiful, free online graphing calculator. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. Quadratic Inequalities. Save Copy. Log InorSign Up. x 2 − 5 x − 6. 1. x 2 − 5 x − 6 > 0. 2. x 2 − 5 x. To solve a quadratic inequality ax² + bx + c > d: Draw the line y = d. Determine the points where the parabola ax² + bx + c crosses/touches this line. To find them, solve ax² + bx + (c - d) = 0. If there's no solution, your parabola is entirely above or below the line. Graph the parabola, taking into account the results from Step 2: The arms. The graph will highlight in green the regions where the graph is above or below zero. Hide/unhide the folders above to switch between the two inequalities. Use the sliders to adjust the values of a, b and c in the quadratic. The red lines will highlight the values of x which correspond to the inequality. You can use the quadratic equation to find the endpoints of the intervals that will be you solution, and would then need to test in which of those intervals the inequality is true. So in this case you could use it to find -5 and 2 [ (-3 +- Sqrt (9+4 (10)1))/2 = (-3 +- 7)/2 = -10/2 or 4/2]. This breaks up the number line into 3 intervals {x<-5.
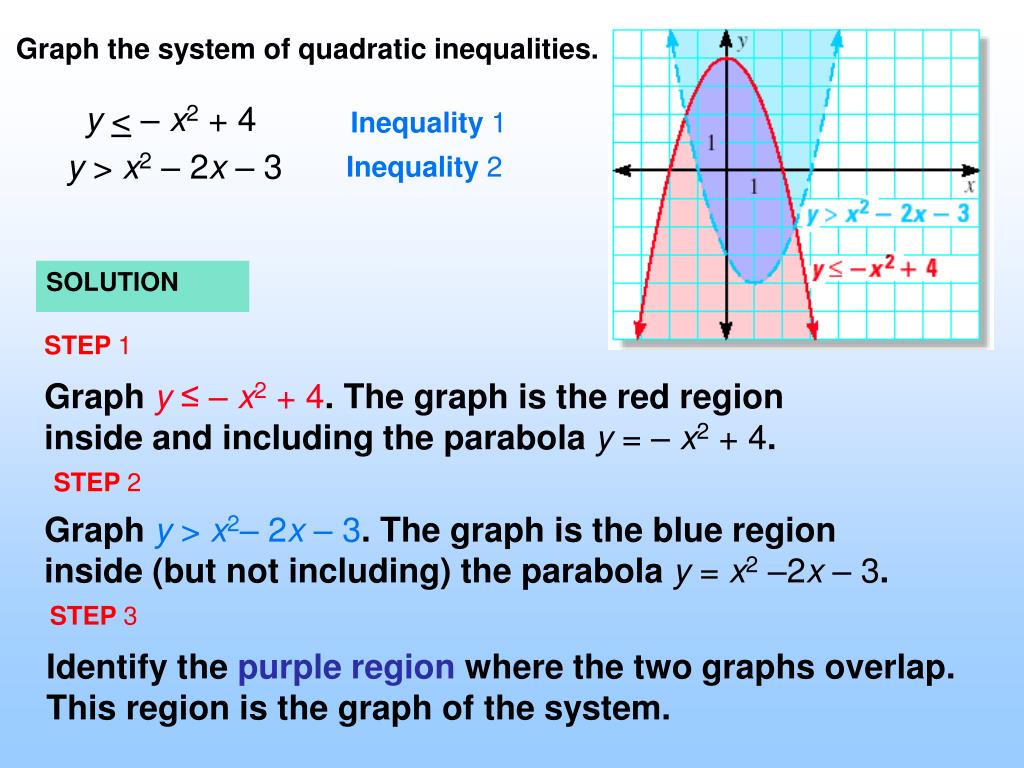
PPT Graphing & Solving Quadratic Inequalities 5.7 PowerPoint Presentation ID6810018
It is important to note that this quadratic inequality is in standard form, with zero on one side of the inequality. Step 1: Determine the critical numbers. For a quadratic inequality in standard form, the critical numbers are the roots. Therefore, set the function equal to zero and solve. − x2 + 6x + 7 = 0. The same basic concepts apply to quadratic inequalities like $$ y x^2 -1 $$ from digram 8. This is the same quadratic equation, but the inequality has been changed to $$ \red $$. In this case, we have drawn the graph of inequality using a pink color. And that represents the graph of the inequality. Write the quadratic inequality in standard form. Graph the function \(f(x)=ax^{2}+bx+c\). Determine the solution from the graph. In the last example, the parabola opened upward and in the next example, it opens downward. In both cases, we are looking for the part of the parabola that is below the \(x\)-axis but note how the position of the. I make short, to-the-point online math tutorials. I struggled with math growing up and have been able to use those experiences to help students improve in ma.
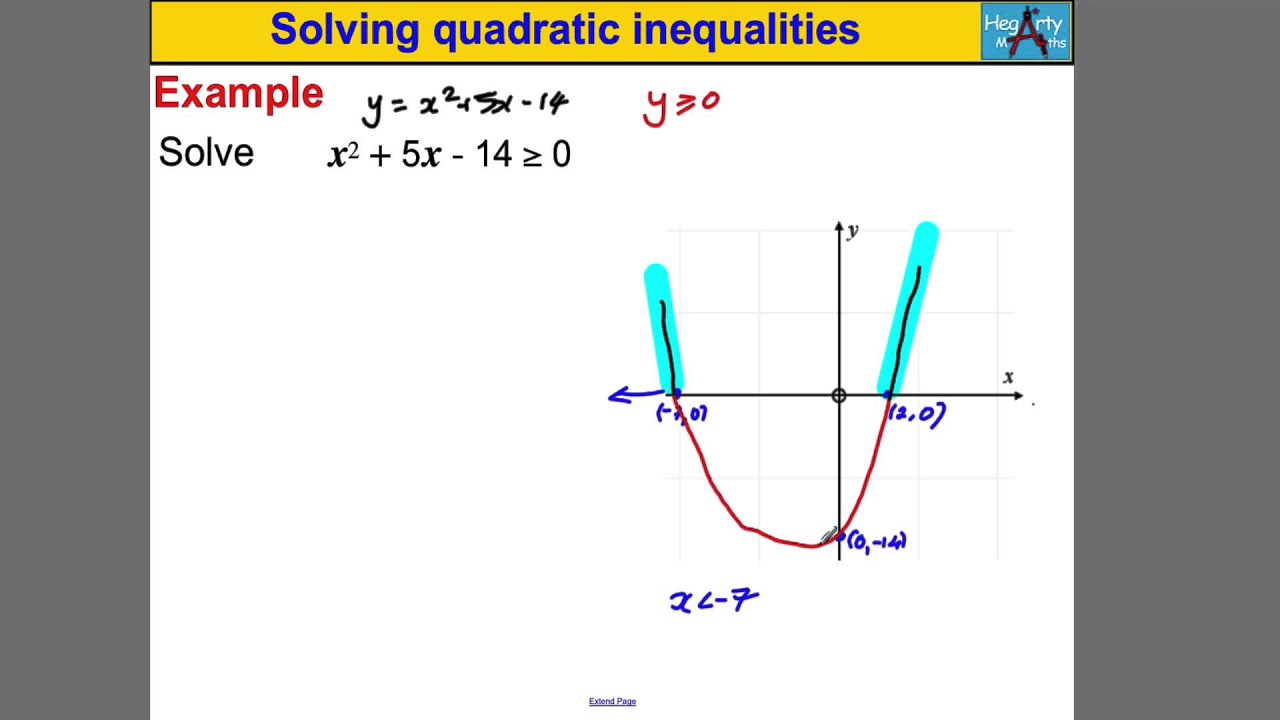
Sketching Graph Quadratic Inequality
Step by step guide to Graphing Quadratic inequalities. A quadratic inequality is in the form \(y>ax^2+bx+c\) (or substitute \(<,≤,\) or \(≥ \) for \(>\)). To graph a quadratic inequality, start by graphing the quadratic parabola. Then fill in the region either inside or outside of it, depending on the inequality. Choose a testing point and. The second inequality is y is less than 2x minus 5. So if we were to graph 2x minus 5, and something already might jump out at you that these two are parallel to each other. They have the same slope. So 2x minus 5, the y-intercept is negative 5. x is 0, y is negative 1, negative 2, negative 3, negative 4, negative 5.
A quadratic inequality is one that includes an x^{2} term and thus has two roots, or two x-intercepts. This results in a parabola when plotting the inequality on a coordinate plane. Solving an inequality means finding the values of x that. The Graph of a Quadratic Function. A quadratic function is a polynomial function of degree 2 which can be written in the general form, f(x) = ax2 + bx + c. Here a, b and c represent real numbers where a ≠ 0 .The squaring function f(x) = x2 is a quadratic function whose graph follows. Figure 6.4.1.

Defining quadratic inequalities and graphing their intervals
The difference between a quadratic equation and a quadratic inequality is that the quadratic equation is equal to some number while quadratic inequality is either less than or greater than some number. Some examples of quadratic inequalities in one variable are: + x - 1 > 0. + 2x - 1 < 0. The standard form of quadratic inequalities in one. This is a cubic equation (the highest exponent is a cube, i.e. x 3), and is hard to solve, so let us graph it instead: The zero points are approximately: −1.1; 1.3; 2.9; And from the graph we can see the intervals where it is greater than (or equal to) zero: From −1.1 to 1.3, and; From 2.9 on; In interval notation we can write:




