To find the area of two similar shapes we can use the knowledge that the ratio of their areas is equal to the ratio of the square of their respective sides. Let us take an example; ABC \ (\sim\) DEF So their corresponding parts must be proportional \ (\frac {AB} {DE}=\frac {AC} {DF}=\frac {BC} {EF}\) Similar figures are identical in shape, but not necessarily in size. A missing length, area or volume on a reduction/enlargement figure can be calculated by first finding the scale factor..
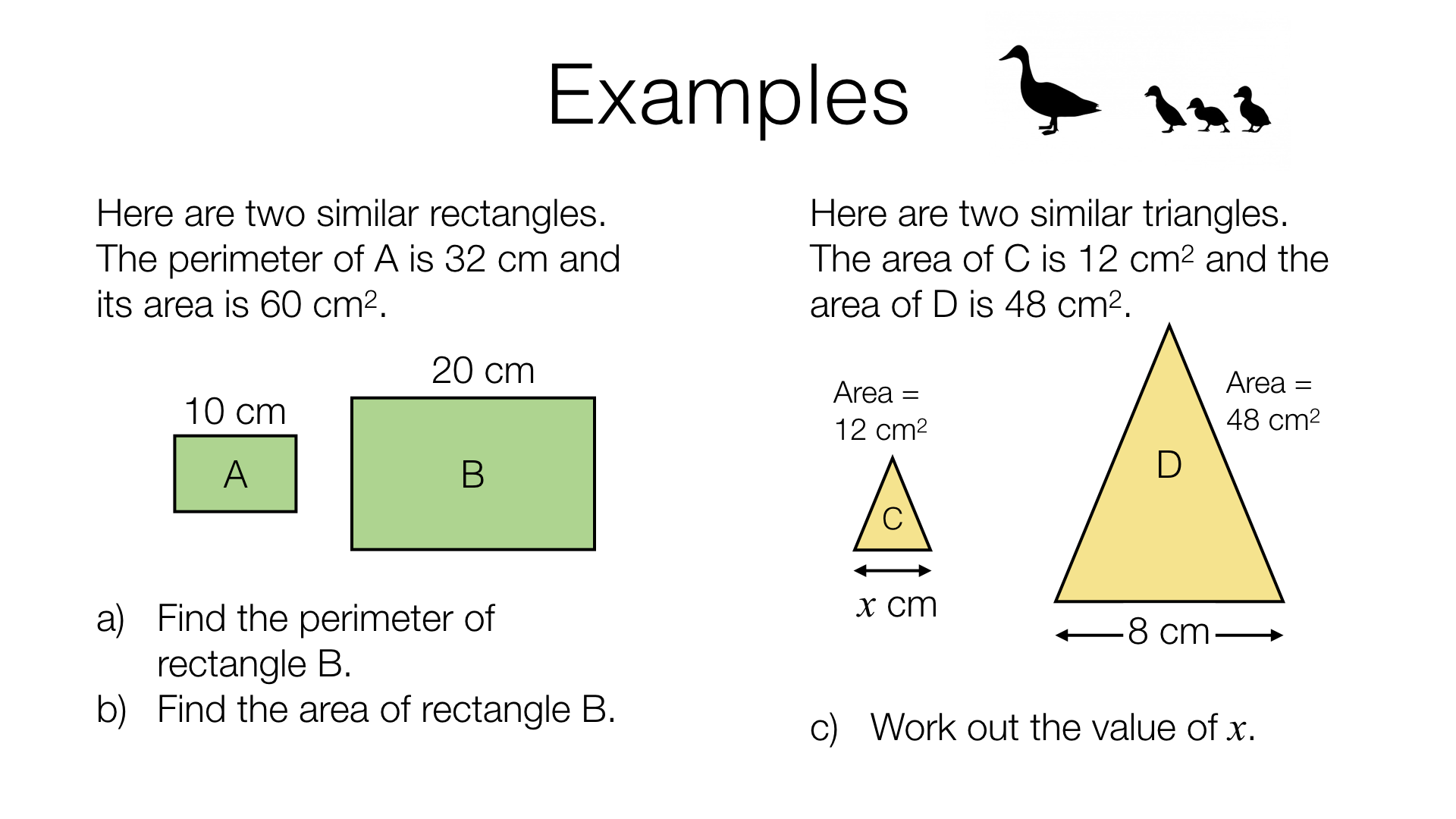
G19a Lengths, areas and volumes in similar shapes
This video explains how areas are connected for similar shapes. It also covers surface areas. What are similar shapes? Similar shapes are enlargements of each other using a scale factor. All the corresponding angles in the similar shapes are equal and the corresponding lengths are in the same ratio. E.g. These two rectangles are similar shapes. The scale factor of enlargement from shape A to shape B is 2 2. The angles are all 90^o 90o GCSE Maths revision tutorial video.For the full list of videos and more revision resources visit www.mathsgenie.co.uk. A video revising the techniques and strategies for looking at similar shapes with area and volume. (Higher Only).This video is part of the Ratio & Proportion.
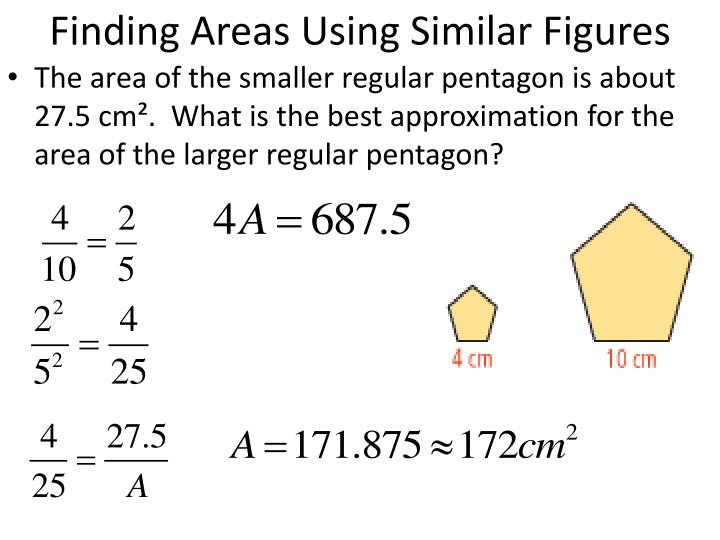
PPT 10.4 Perimeters and Areas of Similar Figures PowerPoint Presentation ID6031832
Area of Similar Shapes How to measure Volume of Similar Shapes How to measure Solved Examples : Key Facts and Summary Recommended Worksheets Introduction Similar shapes are the shapes that have corresponding sides in proportion to every alternative and corresponding angles adequate to each other. Unit 1 Lines Unit 2 Angles Unit 3 Shapes Unit 4 Triangles Unit 5 Quadrilaterals Unit 6 Coordinate plane Unit 7 Area and perimeter Unit 8 Volume and surface area Unit 9 Pythagorean theorem Unit 10 Transformations Unit 11 Congruence Unit 12 Similarity Unit 13 Trigonometry Unit 14 Circles Unit 15 Analytic geometry Unit 16 Geometric constructions The Relation between the Area of Similar Figures. When two figures are similar, the square of the ratio of their corresponding side lengths equals the ratio of their area. When the ratio of two corresponding sides (or other lengths) is expressed as \ (\frac {a} {b}\), in similar figures, the ratio of the areas is expressed as \ (\frac {a^2} {b^2}\) The Corbettmaths video tutorial on Similar Shapes - Areas. Videos, worksheets, 5-a-day and much more

Similar Shapes Length, Area, Volume Bingo Teaching Resources
Start Lesson Back In this lesson, we will identify similar shapes and subsequently work out areas of similar shapes using our knowledge of scale factors. Solution: Linear scale factor = 4 Area scale factor = 42 ( = 16 ) New surface area: 3.5 × 16 = 56 m2 Volume scale factor: If we take a cuboid of length 3 cm and breadth 2 cm 4 cm and height 4 cm then the 3 cm 2 cm Volume = 3 × 2 × 4 = 24 cm3.
Videos, worksheets, 5-a-day and much more. Menu Skip to content. Welcome; Videos and Worksheets; Primary; 5-a-day. 5-a-day GCSE 9-1 The ratio of the area of two similar triangles is equal to the square of the ratio of any pair of the corresponding sides of the similar triangles. If two triangles are similar it means that: All corresponding angle pairs are equal and all corresponding sides are proportional.

Similar Shapes (Area and Volume) Teaching Resources
Two solids are similar if they are the same type of solid and their corresponding radii, heights, base lengths, widths, etc. are proportional. Surface Areas of Similar Solids. In two dimensions, when two shapes are similar, the ratio of their areas is the square of the scale factor. A comparable relationship holds in three dimensions as well. Edexcel Transformations - Edexcel Scale factors of similar shapes - Higher Transformations change the size or position of shapes. Congruent shapes are identical, but may be reflected,.




