5.8 Special Right Triangles Worksheet Name: 10. 30 12. 600 11. 13. 450 15. The shortest side of a 300-600-900 triangle is 15. Find the lengths of the other sides. 16. The hypotenuse of a 300-600-900 triangle is 18. Find the lengths of the other sides. 17. One leg of a 450-450-900 triangle is 9. Find the lengths of the other sides. Special Right Triangles Date_____ Period____ Find the missing side lengths. Leave your answers as radicals in simplest form.. uA Zl 6l v 0rhi Cg Dhut1s J tr cexs5eqruvVePdf. b O zM xald 5ex 6wriVt4h E KI An Tf0i hnmi wt2e 4 tG Le io JmVeytOrmyO.5 Worksheet by Kuta Software LLC 9) u v 8 60°.

Special Right Triangles Notes and Worksheets Lindsay Bowden
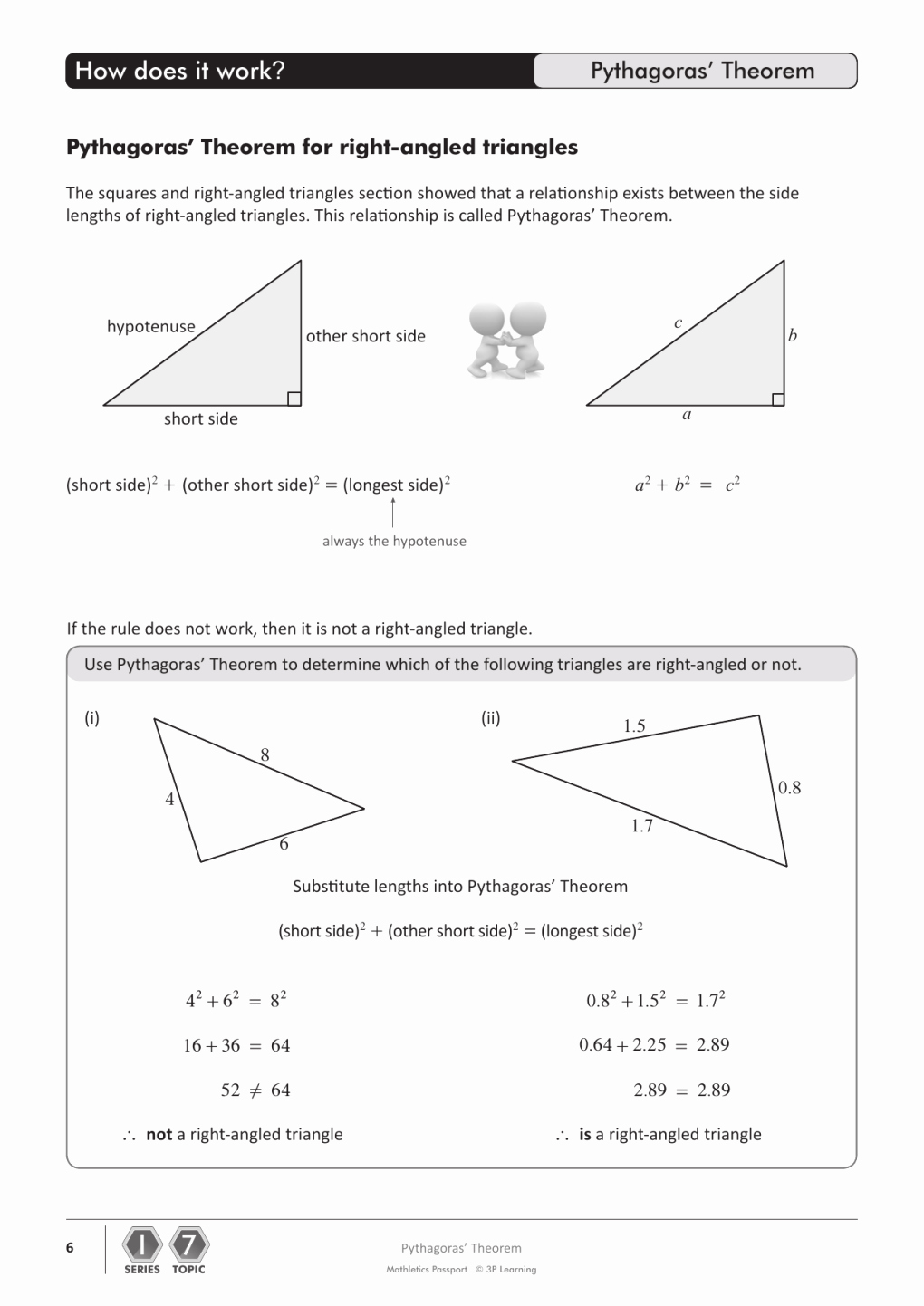
Special Right Triangles Worksheets Tags: 7th Grade Special right triangles are the focus of the below printables. Know the Pythagora's theorem like the back of your hand for nailing these sums. Download PDF Download PDF Download PDF Download PDF Download PDF Download PDF Download PDF Download PDF Download PDF 5-8 Applying Special Right Triangles 1. The sum of the angle measures in a triangle is 180°. Find the missing angle measure. Then use the Pythagorean Theorem to find the length of the hypotenuse. 45°; 2 In a 45°-45°-90° triangle, the legs have equal length and the hypotenuse is the length of one of the legs multiplied by 2 . Special Right Triangles are triangles whose angles are in a particular ratio (30°, 60°, 90° and 45°, 45°, 90°). You can find the right triangle's third side by using the Pythagorean Theorem. It will become more best when you already know the two sides. There are some triangles, such as 45-45-90 and 30-60-90 triangles. Give your answer in simplest radical form. By the Triangle Sum Theorem, the measure of the third angle in the triangle is 45°. So it is a 45°-45°- 90° triangle with a leg length of 8. Example 1B: Finding Side Lengths in a 45o - 45o - 90o Triangle Find the value of x. Give your answer in simplest radical form.
50 5.8 Special Right Triangles Worksheet
Sample Problem 1: What is the length of the hypotenuse of a 45°-45°-90° triangle with leg length √? By the properties of 45°-45°-90° triangle, 30°- 60°- 90° Special Right Triangle In this triangle, one angle is 90 degrees, one is 30 degrees and one is 60 degrees. Properties of a 30°- 60°- 90° Triangle Fortunately, special right triangles worksheets are handy tools for teaching kids all about this topic. But what do these worksheets contain? Let's find out. Right Triangle Trig Worksheet Right Triangles Worksheets 45-45-90 Triangle Worksheet Special Right Triangle Worksheet Right Triangle Worksheet Special Right Triangles Practice Worksheet Multi-Step Special Right Triangles Date_____ Period____ Find the missing side lengths. Leave your answers as radicals in simplest form. 1) 10 45° x 45° 5 2) 7 45° x 45° 14 3) 9 45° x 45° 18 4) 45° 9 x 45° 9 2 5) 45° 5 2 x 45° 5 2 2 6) 9 6 45° x 45° 9 6 2 7) 60° 9 x 60° 9 3 4 8) 5 60° x 60° 5 3 3-1- Special Right Triangles Investigation Worksheet By Peter Jonnard This worksheet is available free to all Teach Simple members. Students are asked to find the missing angles and sides on each type of special right triangle with the given information.

5 8 Special Right Triangles Worksheet 8 Special Right Triangles Pdf
Level: Grade 10 Advanced. Language: English (en) ID: 1684180. 26/11/2021. Country code: AE. Country: United Arab Emirates. School subject: Math (1061955) Main content: Special Right Triangles (2086185) Special Right Triangles. Showing top 8 worksheets in the category - Special Right Triangle. Some of the worksheets displayed are Find the missing side leave your answers as, Special right triangles work name, Find the value of x and y in each 5, 8 multi step special right triangles, Geometry, Reteach applying special right triangles, Geometry practice and homework name special right, Unit 8 right triangles name per.
Our conclusions about triangles ABC and DEF suggest the following theorem: Theorem 4.5.1. In the 30 ∘ − 60 ∘ − 90 ∘ triangle the hypotenuse is always twice as large as the leg opposite the 30 ∘ angle (the shorter leg). The leg opposite the 60 ∘ angle (the longer leg) is always equal to the shorter leg times √3. Special Right Triangles Extra Practice Find the missing side lengths. Leave your answers as radicals in simplest form. 1) m 3 6 n 30° 2) x 3 y 45° 3) u v 3 5 45° 4) m 2 2 n 45° 5) x 10 y 45° 6) 2 x y 60° 7) x y 4 30° 8) x 7 3 2 y 60° 9) 485 x y 45° 10) x 264 y 45°

5.8 Special Right Triangles Worksheet Kayra Excel
58 Special Right Triangles Worksheets - there are 8 printable worksheets for this topic. Worksheets are Find the missing side leave your answers as, 5. And 90° ÷ 2 = 45, every time. If Side 1 was not the same length as Side 2, then the angles would have to be different, and it wouldn't be a 45 45 90 triangle! The area is found with the formula: area = 1 ⁄ 2 (base × height) = base 2 ÷ 2. The base and height are equal because it's an isosceles triangle. Side 1 = Side 2.




