Math Geometry (all content) Unit 4: Triangles About this unit You probably like triangles. You think they are useful. They show up a lot. What you'll see in this topic is that they are far more magical and mystical than you ever imagined! Triangle types Learn Classifying triangles Classifying triangles by angles Theorem 1: The sum of all the three interior angles of a triangle is 180 degrees. Suppose ABC is a triangle, then as per this theorem; ∠A + ∠B + ∠C = 180° Theorem 2: The base angles of an isosceles triangle are congruent. Or The angles opposite to equal sides of an isosceles triangle are also equal in measure.

Congruence Theorems Applet
As per the triangle inequality theorem, the sum of the length of the two sides of a triangle is greater than the third side. Observe the figure given above which shows ABC which represents the Triangle inequality property. If a = 4 units, b = 6 units, c = 3 units, let us verify the triangle inequality property as follows: a + b > c ( 4 + 6 > 3) 1) The exterior angle at a given vertex is equal in measure to the sum of the two remote interior angles. These remote interior angles are those at the other two vertices of the triangle. 2) Knowing this, it follows that the measure of any exterior angle is always greater than the measure of either remote interior angle. The Exterior angle theorem of a triangle states that the exterior angle of a triangle is always equal to the sum of the interior opposite angles. Triangle Formulas In geometry, for every two-dimensional shape ( 2D shape ), there are always two basic measurements that we need to find out, i.e., the area and perimeter of that shape. An interior angle is formed by the sides of a polygon and is inside the figure. The 3 interior angles in every triangle add up to 180 ∘ . Example: 48 ∘ 109 ∘ 23 ∘ 109 ∘ + 23 ∘ + 48 ∘ = 180 ∘ Want to learn more about the interior angles in triangles proof? Check out this video. Finding a missing angle
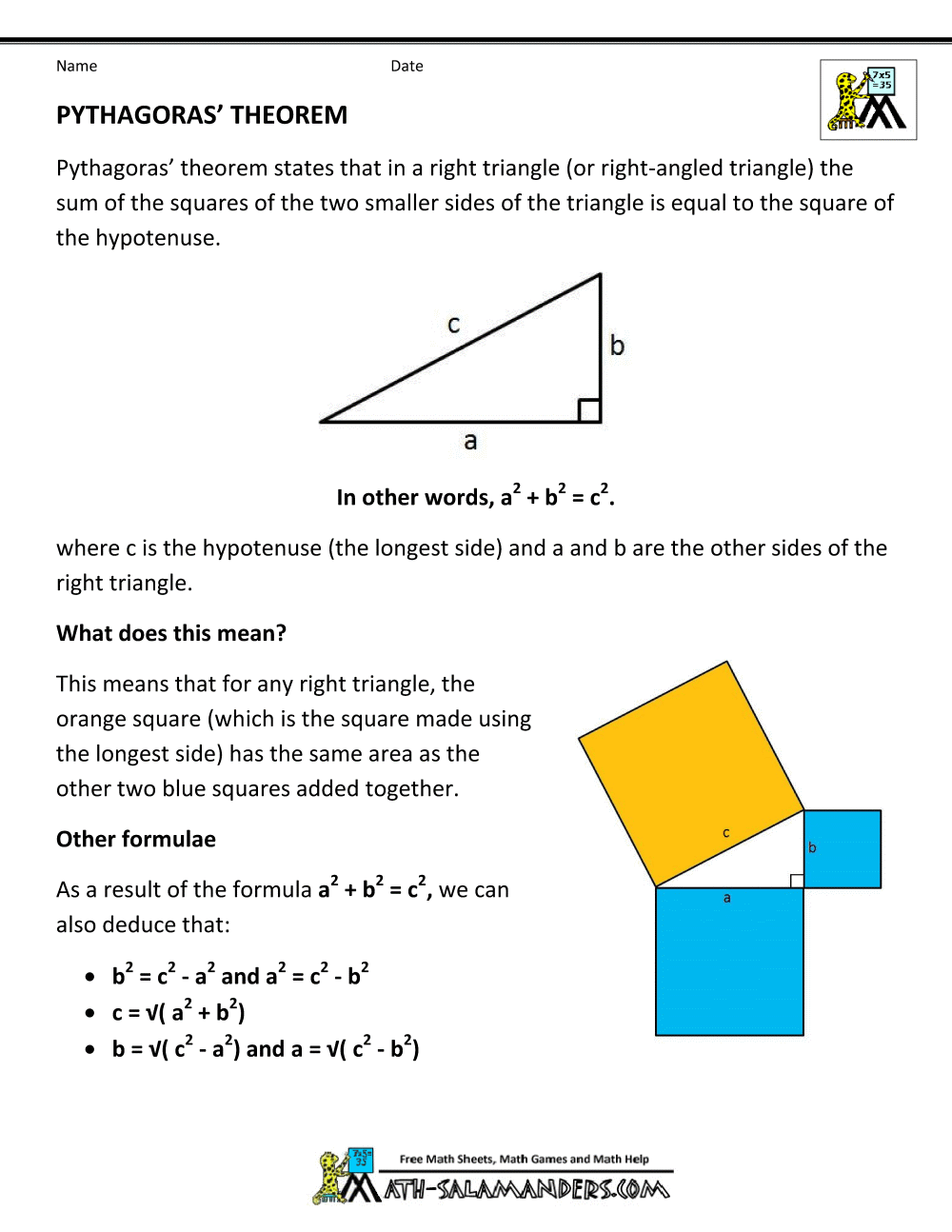
Geometry Formulas Triangles Blog Math 123
The statement "the base angles of an isosceles triangle are congruent" is a theorem.Now that it has been proven, you can use it in future proofs without proving it again. 3. Prove that the measure of an exterior angle of a triangle is equal to the sum of the measures of the remote interior angles. Triangle theorems are based on various properties of this geometrical shape, here are some prominent theorems associated with this is that students must know -. 1. Pythagoras Theorem. Probably the most popular and widely discussed triangle theorems are Pythagoras' one. Pythagoras theorem Class 10 states that 'in a right-angled triangle. Theorem: Triangle Inequality The sum of the lengths of any two sides of a triangle is larger than the length of the other side. This page titled 2.1: Triangles is shared under a GNU Free Documentation License 1.3 license and was authored, remixed, and/or curated by Mark A. Fitch via source content that was edited to the style and standards of. Geometry, You Can Do It! 2 Triangle Classification by angles An acute ∆ is a ∆ with three acute ∠s. An obtuse ∆ is a ∆ with an obtuse ∠s. A right ∆ is a ∆ with a right ∠. An equilateral ∆ is a ∆ with all ∠s ≅. Angle Theorems: Triangles If I asked an entire class to draw a triangle on a piece of paper, then had each person cut out their
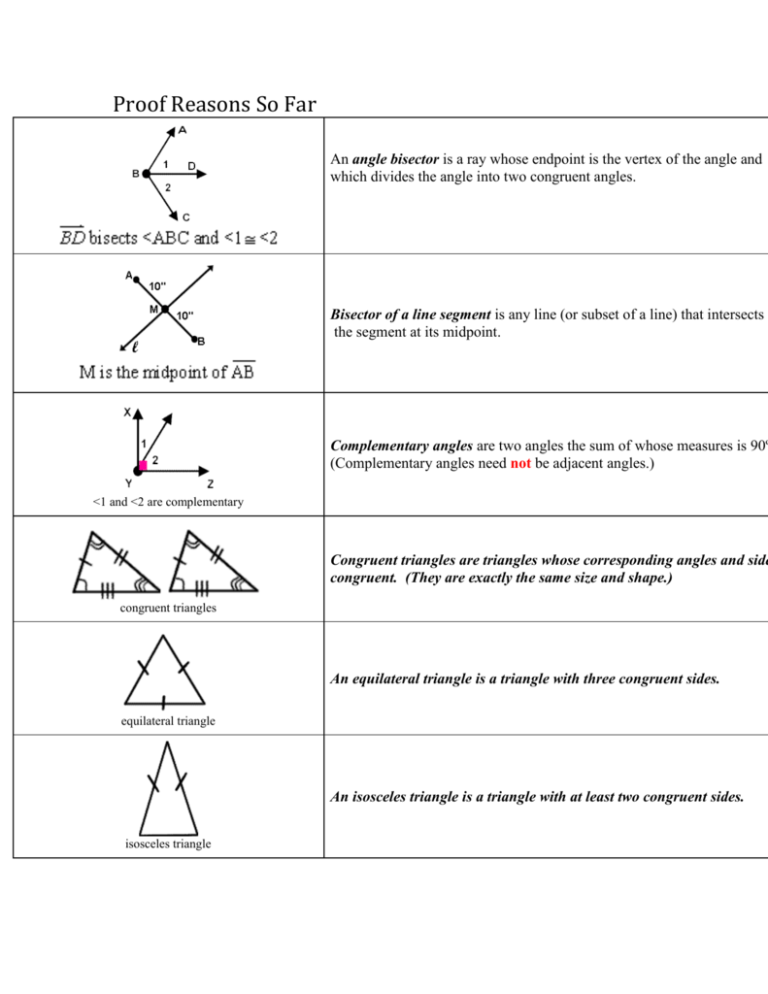
List of Theorems and Keywords so far (Print out)
Geometry Theorems Key components in Geometry theorems are Point, Line, Ray, and Line Segment. Let us go through all of them to fully understand the geometry theorems list. Point In maths, the smallest figure which can be drawn having no area is called a point. Line A straight figure that can be extended infinitely in both the directions Ray Triangles Triangle A triangle is a closed figure in a plane consisting of three segments called sides. Any two sides intersect in exactly one point called a vertex. A triangle is named using the capital letters assigned to its vertices in a clockwise or counterclockwise direction. For example, the triangle below can be named triangle ABC in a
Triangles are one of the most fundamental geometric shapes and have a variety of often studied properties including: Rule 1: Interior Angles sum up to 1800 180 0. Rule 2: Sides of Triangle -- Triangle Inequality Theorem : This theorem states that the sum of the lengths of any 2 sides of a triangle must be greater than the third side. ) Definitions and formulas for the area of a triangle, the sum of the angles of a triangle, the Pythagorean theorem, Pythagorean triples and special triangles (the 30-60-90 triangle and the 45-45-90 triangle) Just scroll down or click on what you want and I'll scroll down for you! Examples of triangles: The area of a triangle:
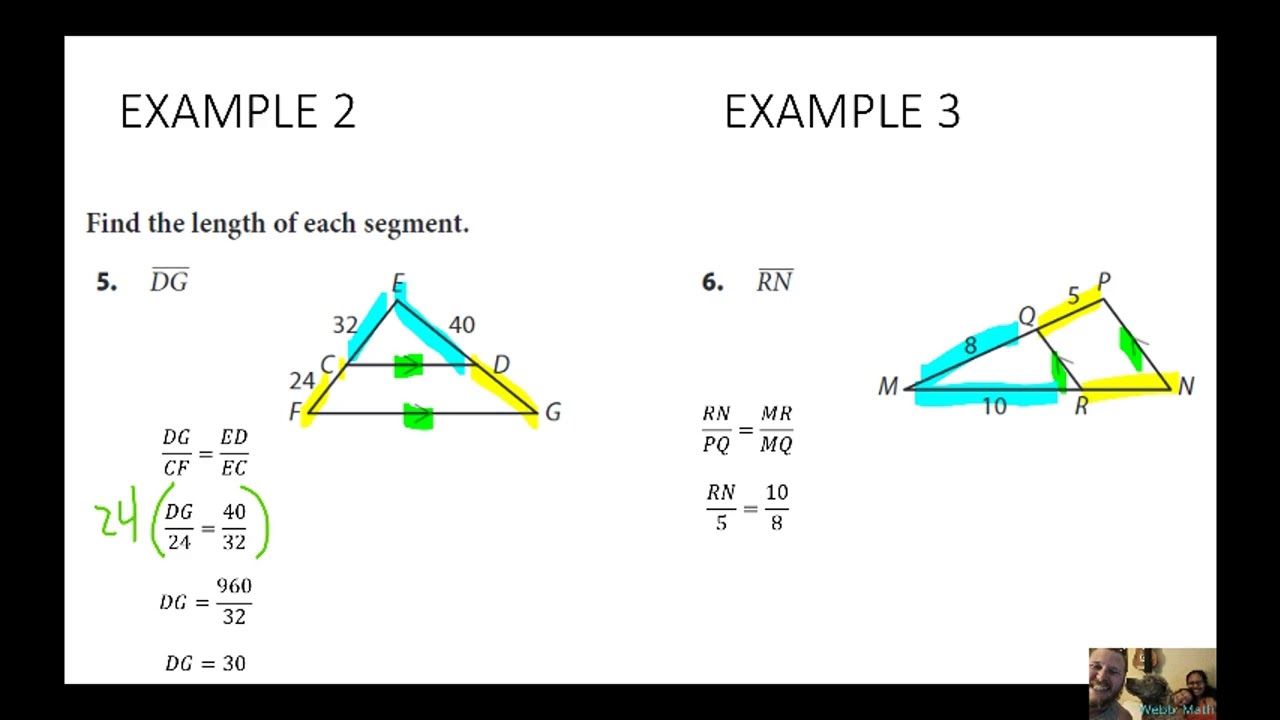
Geometry 12.1 Triangle Proportionality Theorem YouTube
are similar by this theorem because each of their sides are proportional by a factor of 4. is the height of the triangle. Prove that triangle is made up of two congruent triangles, Free practice questions for Common Core: High School - Geometry - Triangle Proofs. Includes full solutions and score reporting. Triangle Proofs. Applications of Triangle Theorems. Find a piece of cardstock or thick paper. Use a ruler and pencil to draw a fairly large random triangle on the paper. Use your ruler to help you to construct the centroid of the triangle. Carefully cut out the triangle and try to balance it on the tip of your pencil.



